Supply-side approaches to the economic valuation of coastal and marine habitat in the Red Sea

1 Introduction

Humans depend upon the natural resources of the Red Sea, including its fish stocks (PERSGA, 2002). Red Sea fish stocks are exploited for both subsistence and commerce. They may also be used for recreation (Gladstone et al., 2012). Economic value is generated by all of these activities.

While humans have been living and using the resources of the Red Sea coast for many millennia, development has become more extensive–even industrialized–in recent years, especially along the Red Sea coast of the Kingdom of Saudi Arabia (KSA). In some cases, this development has led to the degradation of natural fish habitat, including the loss of mangrove wetlands, seagrass beds, and coral reefs (Kotb, 2010; Gladstone et al., 2006). Moreover, coastal development can lead to increased sedimentation due to erosion and to nutrient releases from industrial effluents or sewage disposals. Sediments and nutrient loads affect water quality adversely, thereby further degrading fish habitat (El Sayyed, 2008).

The degradation or loss of natural fish habitat implies losses in economic values. Commercial, subsistence, and recreational fisheries are all affected adversely. In most cases, this loss in value is not measured or anticipated fully, and therefore it is often ignored in decisions to develop the coast. In effect, the ocean habitat, here including coastal wetlands, the seabed and its flora, coral reefs, ocean waters, and the associated ecosystem, is treated as if it may be worthless (i.e., as if it has no price).

Importantly, measures of the economic value generated by fisheries can be used to estimate the value of the ocean habitat that supports fish stocks. These measures are commonly known as “supply-side” or “productivity” approaches to habitat valuation (Barbier, 2007; McConnell and Bockstael, 2005). These measures can be utilized to impute a conservative (lower bound) value on ocean habitat. (This value is conservative because ocean habitat may be a source of value for other ocean uses, including recreation and passive, non-market benefits.) With this information, the value of coastal development can be compared to the value of potentially foregone ocean habitat in order to determine whether the development would be justified economically.

In this paper, we focus on the economic value associated with the exploitation of commercial fish stocks as the most relevant case for the Saudi Arabian portion of the Red Sea. Similar methods may apply to recreational fisheries (Bell, 1997) or, more broadly, to other uses of the ocean or functions of habitat (Barbier et al., 2011). The precise sources and measures of economic value may depend upon the type of use under consideration, however (Barbier et al., 2008).

We describe first the conceptual basis behind supply-side approaches to valuation. Next we review the literature on the use of these methods for valuing ocean habitat. We consider an example based on recent research assessing the bio-economic status of the traditional fisheries of the Red Sea in the Kingdom of Saudi Arabia. Finally, we develop some recommendations for the application of these methods to the Saudi Arabian Red Sea environment.

2 Commercial fisheries and resource rents

The commercial harvesting of fish stocks results in the production of seafood as an economic commodity. Using a commercial fishing technology, sometimes referred to as a “black box,” fishermen produce seafood by combining factors of production, including labor, experience and knowledge, fishing vessels, fuel, nets, bait, ice, and other inputs (Fig. 1). Fish as seafood may be sold as it is or processed for value-added.¹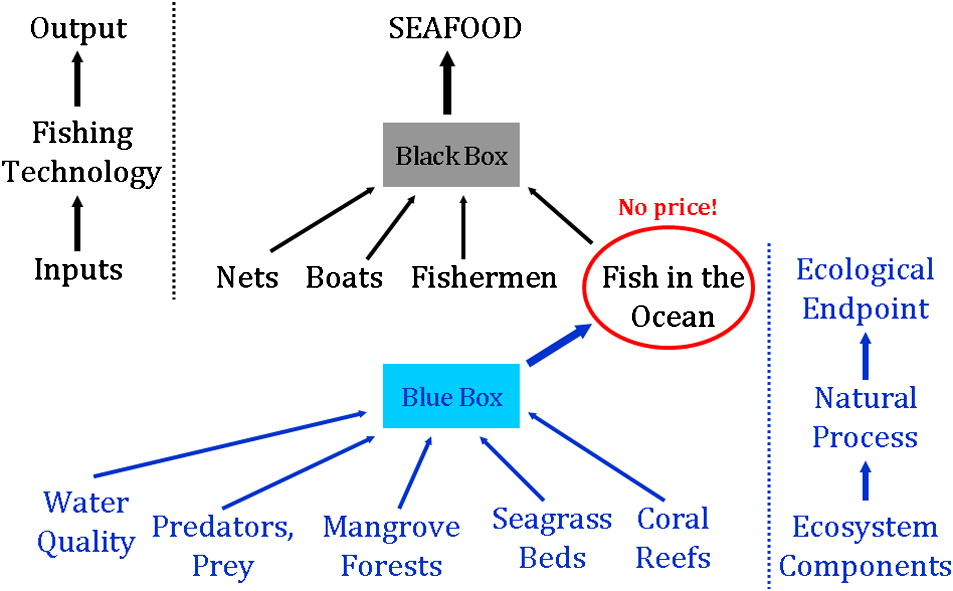

Close

Figure 1

Economic and ecological production functions.

Export to PPT

Economic surpluses result from the harvest of fish stocks. In a well-managed fishery, these surpluses are distributed between fishermen (as producers) and seafood consumers. Taken together, producer and consumer surpluses are the economic measure of value for a commercial fishery (Fig. 2a). Producer surplus is equivalent to the revenues earned from selling catch net of all costs of fishing. Producer surplus is represented by the area below price and above the supply schedule. Consumer surplus is evaluated as what consumers are willing to pay for seafood, less what they actually pay in the market. Consumer surplus is represented by the area below the demand schedule and above price.

Close

Figure 2a

Well-managed (rationalized) fishery with price equal to marginal cost (MC). The market equilibrium occurs at price, p₀, and harvest, h₀. Both consumer surplus (CS) and producer surplus (PS) are realized. If all fishing vessels are identical, in terms of both capital and labor (including fishing skills and knowledge), then the producer surplus comprises the resource rent.

Export to PPT

One element of the producer surplus is known as the “resource rent.” Resource rent is the cost of fish utilized as an input in the production of seafood as a commodity. Resource rent implies that fish have a price, although nature does not charge fishermen this price when fish are removed from their habitat. Because fish both grow and reproduce, the removal of fish from the ocean by harvesting imposes a dynamic cost that depends upon the size of the relevant population; it is this “user cost” that comprises resource rent.² Because this price is not exacted by nature, and it must be estimated by humans, it is sometimes referred to as a “shadow price.”

A fish stock, X, is a renewable resource. The fish stock is capable of growth, i.e., there is a dynamic “flow” of fish, where $\dot{X}$ denotes the change in the stock per unit of time, which depends upon the size of the stock at any point in time: $$\dot{X}=F(X)$$

Typically the growth of the fish stock, F(X), increases at low stock sizes, reaches a peak at a level known as “maximum sustainable yield” (MSY), and decreases until the stock reaches its ecological “carrying capacity.” Fish harvest, h(E,X), is a function of both fishing effort, E,³ and stock size, X, and it removes biomass from the stock: $$\dot{X}=F(X)-h(E\text{,}X)$$

Fish may be harvested from the ecosystem at a cost, c(X), that depends inversely upon the size of the stock. Fish that have been harvested are delivered to a market, where they fetch a price, p(h), which depends upon the demand for and the supply of fish. In steady-state equilibrium, or at any point in time on a path to equilibrium, it is economically optimal to harvest the fish stock so that the discounted sum of net social benefits (i.e., economic surpluses) per period is maximized. Under the optimal condition, the marginal revenue of harvest equals the marginal cost of harvest, c(X), plus the user cost (the resource rent), μ. Thus, for a constant price of fish at the dock, p, the resource rent is: $$\mu =p-c(X)$$

At the economic optimum, fish should be harvested according to the following “modified golden rule”: $$\delta =F_X-\frac{c_{X}F}{\mu }$$

This rule states that the difference between an incremental “investment” in the fish stock in situ, F_X (by letting it continue to grow in the ocean), and the incremental gain from harvesting it now, c_XF/μ, must equal the discount rate, δ (Munro and Scott, 1985).

Because there is no market for fish prior to harvest in their natural environment, in an unregulated fishery, fishermen treat fish as an unpriced input in the production of seafood (Fig. 1). Fishermen fish more than they should because the absence of a price on fish in the ocean is perceived as an implicit subsidy in the production of seafood. In such a situation, competition among fishermen for the fish reduces the stock and increases the cost of fishing so that resource rents dissipate (and therefore μ = 0). This competition leads to excessive fishing effort (too many vessels and too much labor) and drives stocks to levels that are too low from society’s point of view (Fig. 2b).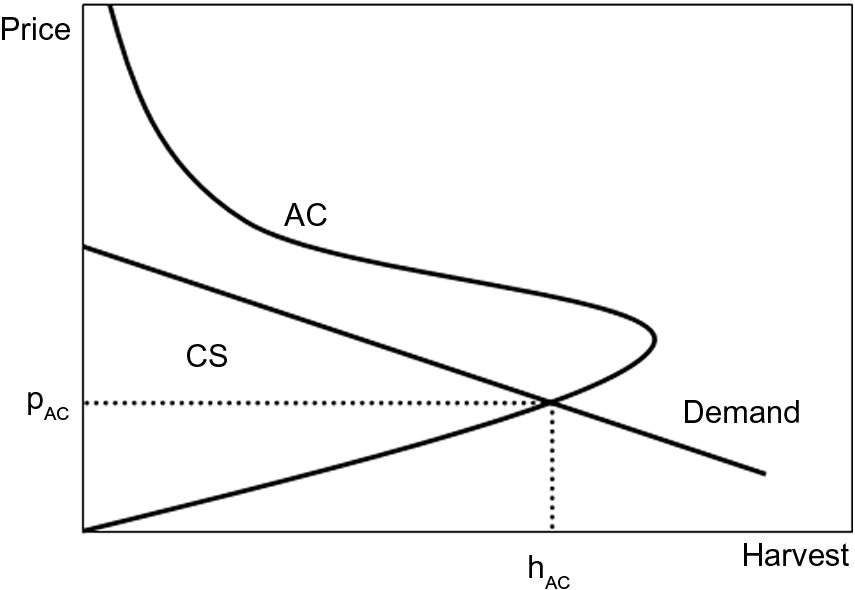

Close

Figure 2b

Open-access fishery with price equal to average cost (AC). The market equilibrium occurs at price, p_AC and harvest, h_AC. Average cost comprises a backward-bending supply curve. There is no producer surplus, and therefore no resource rent, and only consumer surplus (CS) is realized.

Export to PPT

The optimization of economic surpluses requires the implementation of appropriate regulations (often referred to as “conservation and management measures”) in the fishery. Many different types of fishery regulations have been devised, but only a few have the potential to optimize economic surpluses. Economically optimal measures impute a price to the fish, through either the imposition of taxes on harvests or on fishing effort or the assignment of transferable rights in shares of allowable catch (quota rights) or in fishing areas.

Commercially exploited fish stocks depend upon the ocean habitat (or ecosystem), O, where they are found, including wetlands, coral reefs, the seafloor, seagrass beds, water quality, ecological relationships, and sources of food. Analogous to the “black box” representing the economic technology for producing seafood from an array of inputs, we define a “blue box” to represent the ecological “technology” for producing fish in the sea from components comprising ocean habitat (Fig. 1). Indeed, ocean habitat is a sine qua non of the fishery, and the carrying capacity, K, of the environment may be viewed as a positive function of habitat, possibly with diminishing returns: {K(O): K′ > 0, K″ ⩽ 0}. Further, because the growth of the fish stock depends upon the carrying capacity of the ecosystem, growth could be modeled explicitly as a function of ocean habitat as well: F(X,O).

3 Ocean habitat and ecosystem services

Because ocean habitat supports commercial fish stocks, ocean habitat can be conceived as another type of input in the production of seafood. Under this interpretation, ocean habitat provides what is often referred to as an “ecosystem service.” In theory, ocean habitat can be partitioned into its component parts, and each of these components can be interpreted to provide an ecosystem service. Typically, there is much uncertainty about the blue box technology, i.e., how these components interact to produce the composite ocean habitat. Further, there may be either natural or human-induced variations in the components. Finally, there may be inexact measures of the ecological contribution of each of these components (i.e., early studies modeled wetland acreage as the only relevant proxy for habitat, and even some modern studies find a similar result (Aburto-Oropeza et al., 2008)).

In theory, the economic surpluses that result from the commercial harvesting of fish can be imputed to the ocean habitat in aggregate (or to its components individually), thereby assigning an economic value to the ocean habitat as an ecosystem service. This method of valuing the ecosystem services of ocean habitat is called the “productivity approach”.⁴ It is appropriate to impute both producer and consumer surpluses in the downstream seafood markets to measure the value of ecosystem services. In practical applications, however, because of data limitations or ecological uncertainties, often only a portion of surpluses, such as resource rents, are used as a measure of value. Finally, value can be imputed either to the fish stock, the ocean habitat supporting the fish stock, or the individual components comprising ocean habitat. Assigning value to more than one of these three types of services simultaneously would lead to a double-counting of values.

The productivity approach is a supply-side method for valuing ecosystem services (Ellis and Fisher, 1987). It should be contrasted with other valuation approaches that focus on the willingness-to-pay (WTP) for the non-marketed attributes of the ocean ecosystem.⁵ WTP approaches include the travel cost, hedonic pricing, and contingent valuation methods of estimating the benefits to individuals or firms. In situations where a total economic value (TEV) is being estimated, it may be appropriate to apply multiple valuation methods, such as combining the productivity and WTP approaches.⁶ DeGroot et al. (2012) review and summarize recent estimates of TEVs for ecosystem components, including those for open oceans, coral reefs, coastal systems, and coastal wetlands, emphasizing the uncertain and contextual nature of the estimates.

In developing a productivity measure of the value of ocean ecosystem services, it is important to include all of the fisheries that are supported by the ocean habitat. The development of such a comprehensive productivity measure raises questions about the optimal mix of fisheries, where gear conflicts, bycatch, or other external costs may arise among disparate fishery sectors or fleets.

In the prototypical case in which market price is unaffected by supply from the fishery, the use of supply-side measures to value the ecosystem services provided by ocean habitat depends crucially upon whether fish are managed very well. If the fishery is unregulated, and the fish are thereby overexploited, the value imputed to the habitat would be diminished considerably. In such a case, economic surpluses would be limited to those accruing only to the more skilled fishermen (so-called “highliner” rents). Where fishermen are similar in terms of skills, technology, and behavior, theory suggests that resource rents would be dissipated completely. Consequently, the imputed value of the habitat would be close to zero (cf., Lynne et al., 1981). Where a fishery is regulated to maximize the net social benefit, the value of the ocean habitat supporting the fishery is also thereby maximized.

The ocean can be of varying quality for fishery habitat, depending upon its natural characteristics, the nature of ecological relationships, and the extent of human influences. Although it is obvious that the characteristics of habitat provide support for fish stocks, in many cases the physical, chemical, and ecological relationships between the habitat and the growth and maintenance of fish stocks are poorly understood. Consequently, it can be problematic to understand how changes in habitat lead to changes in fish stocks (Armstrong and Falk-Peterson, 2008).

In many applications, stakeholders and decision-makers are interested in estimating the economic damages associated with changes to habitat. For example, the loss of wetlands due to coastal development could lead to changes in the stock size of fisheries that depend upon the wetlands as nurseries, for protection from predators, or as a source of food. In these situations, it is critically important to understand the physical, chemical, and ecological relationships. Further, there may be value to undertaking scientific research that uncovers the nature of these relationships (Barbier et al., 2008).

4 Consumer surplus in seafood markets and distributional effects

Where the demand for fish is inelastic, it is important to consider the distributional effects of management measures. Freeman (1991) has shown that there could be a larger surplus gain from the improvement of habitat in an open-access fishery than in a well-managed fishery. In seafood markets where price is inelastic, an increase in habitat shifts both average and marginal costs down. In the open-access case, average cost provides the relevant supply schedule. There are no resource rent gains in this case, because rents have been dissipated completely. Consequently, only consumer surplus gains are realized as price decreases when average cost shifts down (Fig. 3a). On the other hand, in the well-managed fishery, marginal cost is the relevant supply schedule. There are surplus gains to both producers and consumers (a portion of consumer surplus gains actually are a transfer from producers and therefore do not count as a social gain) (Fig. 3b). Freeman shows that it is theoretically possible that the gains to consumer surplus in the open-access fishery exceed the combined gains to producer and consumer surpluses in the well-managed fishery.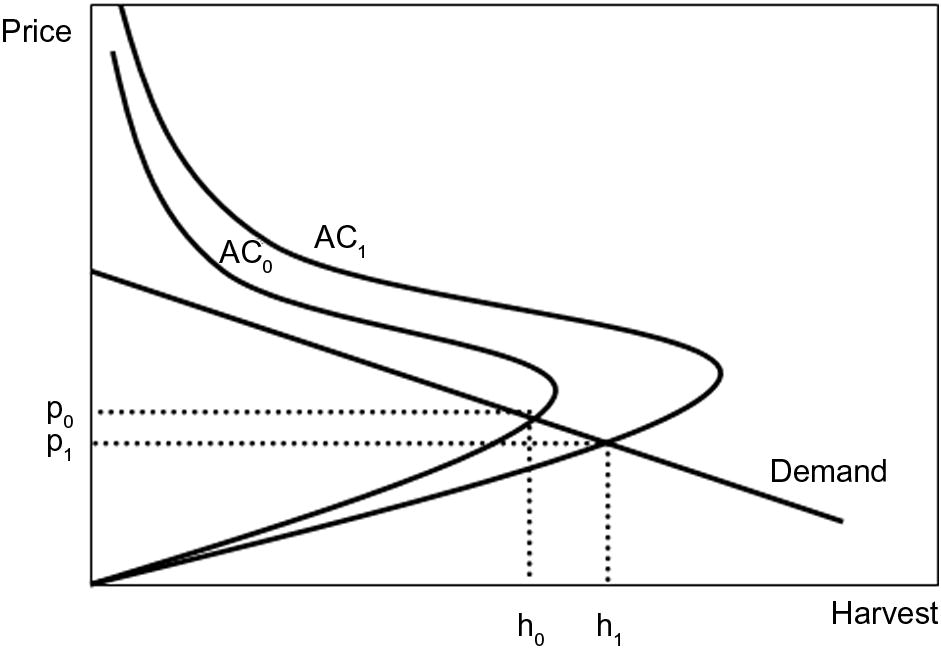

Close

Figure 3a

Open-access fishery with price equal to average cost, as in Fig. 2b. The initial market equilibrium occurs at price, p₀ and harvest, h₀. Improvements in ocean habitat lead to an expansion of the target fish biomass, reflected in a shift of average cost to the right, from AC₀ to AC₁. The new market equilibrium occurs at p₁ and h₁, where yields are greater and price is lower. Even with these shifts, which could lead to a larger consumer surplus, there is no resource rent.

Export to PPT

Close

Figure 3b

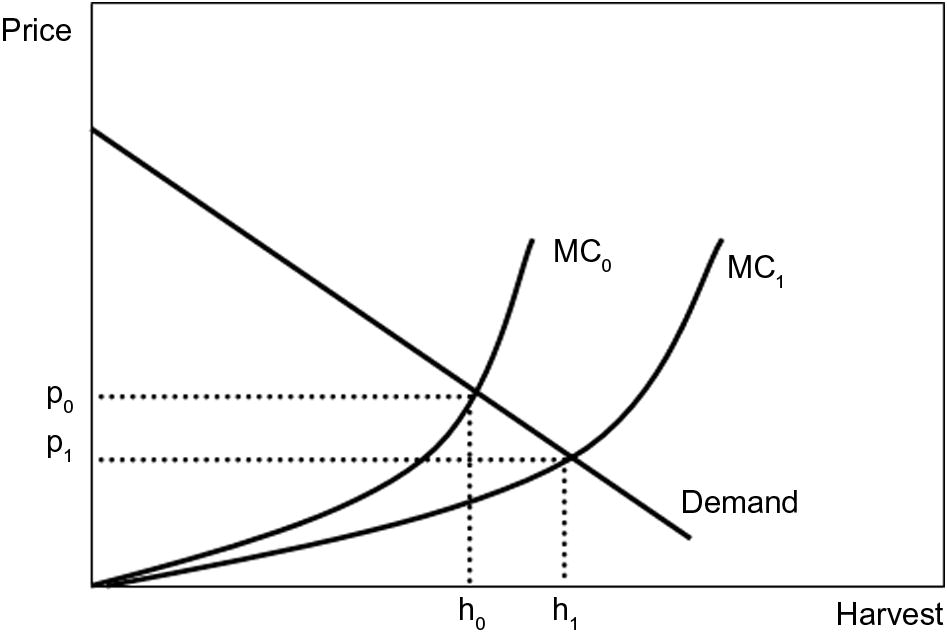
Well-managed (rationalized) fishery with price equal to marginal cost (MC), as in Fig. 2a. The initial market equilibrium occurs at price, p₀ and harvest, h₀. Improvements in ocean habitat lead to an expansion of the target fish biomass, reflected in a shift of marginal cost to the right, from MC₀ to MC₁. The new market equilibrium occurs at p₁ and h₁, where yields are greater and price is lower. At the new equilibrium, there are increases in combined consumer and producer surpluses, resource rents are realized, and there is a partial transfer of surpluses from producers to consumers.

Export to PPT

Thus, in the case of a commodity where demand is relatively unresponsive to price changes (i.e., there may be few close substitutes), there may be significant gains from improvements in habitat. As a corollary to this finding, there may be larger losses (again to consumer surplus) from the degradation of habitat in the open-access case with inelastic demand. Consequently, in such a situation, a policy to manage fisheries to maximize economic yield should lead to smaller losses relative to open-access when faced with impending or ongoing habitat degradation.

McConnell and Strand (1989) consider a different (“demand side”) case where seafood demand is affected by water quality. They show that, in the open-access case, where consumer surplus is the only relevant measure of welfare, improvements in water quality in theory could expand demand to the point where the fishery is seriously overexploited, raising price and lowering harvests. In the extreme, improvements in water quality could actually decrease welfare (Fig. 4). The corollary to this finding suggests that the loss of ocean habitat, as measured by the degradation of water quality, could lower seafood quality, thereby contracting demand, and improve welfare as price drops and harvests increase.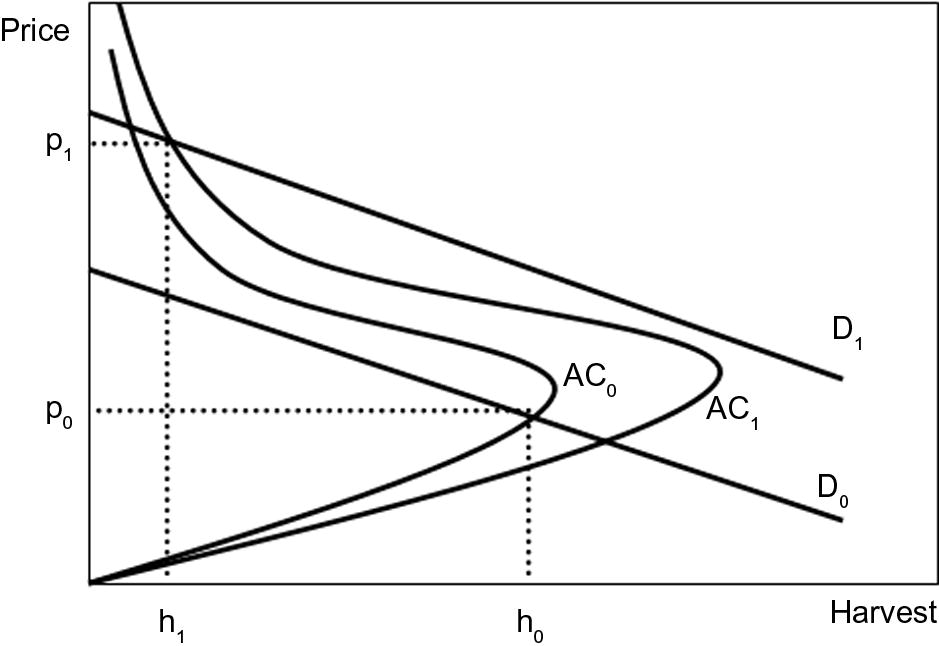

Close

Figure 4

An open-access fishery with an expansion of demand due to an increase in seafood quality in combination with improvements in ocean habitat. (Note that higher seafood quality could be a consequence of the habitat improvements.) The initial market equilibrium occurs at price, p₀ and harvest, h₀. Demand expands from D₀ to D₁ because of higher seafood quality, and average cost shifts to the right, from AC₀ to AC₁, due to habitat improvements. The new market equilibrium occurs at p₁ and h₁, where yields are much lower and price is much higher. Consumer surpluses (the only source of value in an open-access fishery), reflected after the shifts by the area below D₁ and above p₁, has declined significantly as a consequence of environmental improvements.

Export to PPT

5 Valuing ecosystem services

Some of the earliest efforts at valuing ecosystem services are now widely regarded as methodologically inadequate or even invalid. Among these were efforts to estimate the energy flow through wetlands using an energy theory of value (Gosselink et al., 1974).⁷ Nevertheless, these early efforts served an important purpose in motivating economic research on the productivity approach. In this section, we examine three supply-side approaches to valuing ecosystem services, and, in the next section, we present an example of the use of these approaches for the traditional fisheries of the Red Sea. To enhance comparisons, all value estimates in this paper have been adjusted to 2012 US dollars, using an exchange rate of SAR3.75/$1.00 and deflating with the US Consumer Price Index.

6 An example: the traditional fisheries of the Red Sea

Jin et al. (2012) apply standard bioeconomic methods to characterize the current status and the potential of the traditional fisheries off the northern and central regions of the Red Sea coast of the Kingdom of Saudi Arabia (KSA). (These two regions can be distinguished ecologically from a southern region, for which statistics are harder to compile.) In these mainly artisanal coastal fisheries, fishermen deploy hand-lines, gillnets, and traps from small, open motorboats. They catch and land groupers, snappers, emperors, barracudas, scads/jacks/trevallies, kingfish, and tunas. These species are known to spend significant portions of their lifecycles in association with the coral reefs along the coast, and thus estimates of the value of the fisheries could be used to impute values to the services provided by the coral reef system.¹⁴

Using time-series data on fishing effort (boat-days) and landings obtained from the KSA Department of Marine Fisheries, the authors apply a Clarke–Yoshimoto–Pooley approach to estimate the collective intrinsic growth rate, r, carrying capacity, K, and catchability, q, for these seven stocks. With these parameters and with calculations of a weighted average price across the seven target species and of fishing costs, the authors calculated actual open-access (OA) yields and theoretical maximum sustainable and economic yields (MSY and MEY).

The authors found that current annual fishing effort levels, ranging between 300,000 and 350,000 boat-days, constituted clear over-fishing of the stocks. Exploitation at these levels resulted in combined OA yields of 7725 mt, leaving standing stocks of 6440 mt. Total revenues were estimated to be $46 million, but net social benefits were estimated to be zero. In contrast, using a 5% rate of discount, the authors calculated a socially optimal level of fishing effort at 146,000 boat-days. Optimal exploitation at this much reduced level would result in MEY yields of 8901 mt with standing stocks of 18,644 mt. Total revenues at MEY were estimated to be $53 million, with net social benefits of $35 million. The authors also calculated yields, standing stocks, revenues, and resource rents for MSY (Table 2).

Using these estimates, we can approximate the ecosystem service values of the coral reef-seagrass bed systems (Table 2).¹⁵ Ideally, one would like to model the blue-box technology from Fig. 1 directly, given that many factors likely contribute to the biological productivity of the Red Sea fish stocks, including water quality (temperature, salinity, transparency, and nutrient levels), mangrove cover, seagrass beds, and coral reefs, among others. Further, these factors may vary geographically and over time in terms of their relative contributions to fishery productivity. Without detailed information on the blue-box technology and its spatial and temporal variability, for expositional purposes, we assume that the most important ecosystem service is a homogeneous and unchanging coral reef-seagrass complex. For the northern and central regions combined, the area of this complex has been estimated to be 5000 km².¹⁶

The calculations of annual per-unit ecosystem service values are straightforward. Using the total value approach, we divide gross revenues by the area of the coral reef-seagrass bed complex to obtain the annual per-unit value. We do the same thing with estimated resource rents to obtain the annual per-unit value for the residual rent approach. The marginal productivity approach requires an assumption about the relationship between yields and ocean habitat. Assume that carrying capacity is directly proportional (here equal) to ocean habitat, K∼O. Jin et al. (2012) apply the following Fox-type production function to the fishery: $$h={\mathit{qEKe}}^{-q/\mathit{rE}}$$

Letting K = O, and taking the derivative with respect to O gives: $$\frac{\mathit{dh}}{\mathit{dO}}={\mathit{qEe}}^{-q/\mathit{rE}}$$ and the marginal productivity measure is calculated as: $${\text{MVP}}_O=p\frac{\mathit{dh}}{\mathit{dO}}={\mathit{pqEe}}^{-q/\mathit{rE}}$$

Table 2 presents estimates of the imputed per-unit ecosystem service values ($/km²) for the reef-seagrass complex using each of the three methods described above. The ecosystem service values obtained using the “total value” approach are much larger than those obtained with the two other methods. We have discussed already the reasons why these estimates may be inappropriate. Note that with both the total value and the marginal productivity approaches, the MSY estimate exceeds both the OA and MEY estimates. This result is due to the nature of the underlying biological production, ensuring that MSY leads also to the maximum total revenues and marginal values. Under either approach, whether the ecosystem service values at MEY exceed or fall below those at OA depends critically upon the costs of fishing, so that high costs could lead to the (seemingly counter-intuitive) result that OA ecosystem service values exceed those at MEY.

Only when employing the residual rent approach do we obtain the sensible result that ecosystem service values are maximized when the fishery is managed rationally to optimize resource rent. Resource rent is zero under OA. Some level of resource rent is realized at MSY, but this is exceeded by that obtained at MEY. Importantly, MEY leads to an outcome where there is a larger standing stock. Although the existence of higher stock levels has not been explored here, there may be ancillary benefits to recreational fisheries, ecosystem health (say, through the grazing by fish of algae on coral reefs), and insurance against natural or anthropogenic hazards (storms, harmful algal blooms, and pollution events).

In this example, we have assumed that the full resource rent should be imputed to the coral reef-seagrass bed complex; in reality, we would want to specify a blue-box ecological production function, distributing the rent across relevant ecosystem factors. When used in a cost-benefit context, we also should consider capitalized per-unit ecosystem service values (Table 3). Under MEY, capitalized ecosystem values derived from the traditional Red Sea fisheries and summed across all of the relevant coral reefs are on the order of $1 billion, surely rivaling the net benefits of some forms of industrial development.¹⁷ Finally, note that we have estimated ecosystem service values for the traditional fisheries only (a subset of “provisioning services”). There are likely to be significant service values contributing to the industrial and recreational fisheries, recreational diving, passive non-market benefits, and other valued endpoints. While extant estimates of an array of other sources of value, including provisioning, regulating, habitat, and cultural values, are known to be uncertain and dependent upon context, a recent compilation of estimates suggests that provisioning services (interpreted broadly) may account for only about 15% of the ecosystem service values for coral reefs (deGroot et al., 2012).

7 Ecosystem-based management considerations

Our focus has been on ocean habitat, per se. Fishery resources exist within an ecosystem context, and species interactions may be important in determining the productivity of fisheries. In particular, other species, guilds, or trophic levels may be understood to provide ecosystem services, and a full treatment of ecosystem services may require the characterization of ecological interactions. Further, coastal development can be conceptualized as a process of exploiting a non-renewable resource with implications for the health of an associated renewable resource. These interactions fall within the realm of ecosystem-based management.

8 Discussion

Commercial fisheries are just one of many human activities that use ocean habitat in the Red Sea. Red Sea habitat also may be valued passively for its existence, for the use of future generations, or in order to leave options open for future uses. Some human activities may diminish the value of ocean habitat for other activities or passive uses. These include the overexploitation of fish stocks, the pollution of the marine environment through the release of nutrients or the spilling of hazardous materials, and the clearing of mangroves. Some human impacts may be irreversible, such as construction activities in mangrove wetlands or the sedimentation of coral reefs from coastal erosion near developments.

It is important to estimate the value of ocean habitat in order to make rational decisions about the best mix or sequence of coastal and ocean uses. A supply-side approach values ocean habitat using estimates of consumer and producer surplus in downstream markets. In essence, ocean habitat is treated as an input (alternatively, it provides an “ecosystem service”) in the production of commercial goods and services.

We examine supply-side valuation approaches for ocean habitat using commercial fisheries as a relevant example. Similar techniques may be employed to value ocean habitat for subsistence and recreational fisheries. Other methods may be needed for valuing passive uses. In the case of commercial fisheries, typical supply-side valuation involves modeling ecological carrying capacity for fisheries as a function of ocean habitat. Extant studies consider one measure of ocean habitat, such as wetland acreage, the proportion of the seafloor covered by submerged aquatic vegetation, or a water quality index. The most appropriate methodological approach depends upon the availability of data as well as the institutional setting. Because non-market valuation methods also require accurate information on the relevant ecological and economic interactions, the results of supply-side valuation studies can provide insights beneficial to these studies as well.

In order to estimate the value of ocean habitat, data must be compiled on fish landings, fishing effort, seafood prices, and one or more measures of ocean habitat. Statistical estimation requires variation in all of these variables, including the measures of ocean habitat. This variation might be observed in either cross-sectional data, time-series data, or both.

Institutional settings–both market and regulatory–are important. We expect that resource rents will be small or nonexistent in open-access fisheries. Consequently, consumer surplus may be an important measure of economic value. Consumer surplus may be affected when demand for seafood is inelastic or when seafood quality depends upon the condition of ocean habitat (e.g., water quality). Where demand is inelastic, due to the absence of close substitutes—such as in the case of subsistence fisheries, welfare changes may be very sensitive to changes in ocean habitat.

9 Recommendations

The most important recommendation is the need to compile data useful for developing statistical models to estimate the value of ocean habitat. In the Saudi Arabian sector of the Red Sea, data currently are compiled on fish catches, fishing effort, and seafood prices. Additional data are needed to describe variations in ocean habitat across fishing regions and over time.

Measures of ocean habitat include mangrove acreage or coastal coverage, coral reef acreage, water quality, sea grass acreage, among other possibilities. Research on the ecological linkages between measures of habitat and the productivity of fish stocks can help inform the choice of measures for supply-side valuation. This research should include developing a better understanding of the extent to which the life cycles of exploited species depend upon the coral-seagrass-mangrove system. For many commercial species, research in tropical areas outside of the Red Sea environment could help inform the choice of habitat measures.

Once data are available, models must be developed to measure the marginal economic productivity or the resource rents of the Red Sea habitat. These models should be refined and updated on a regular basis as new data become available.

Results of the valuation should be made available for use in decisions about the economic consequences of human activities that may adversely impact the Red Sea habitat. For example, decisions to undertake coastal construction leading to the cutting of mangrove wetlands or the destruction of coral reefs should not be made without consideration of the opportunity costs, in terms of lost habitat productivity.

Decisions about coastal development should be informed by economic analysis, but they must also take into consideration the issues of fairness to existing users and the likely value of the resource to future generations. In cases where the net social benefits of coastal developments are positive, even if they lead to habitat degradation, further consideration should be made of the potential impacts on passive uses of the Red Sea environment. This consideration may require the application of a different set of methodological tools.