Validation study of the reactor physics lattice transport code DRAGON5 & the Monte Carlo code OpenMC by critical experiments of light water reactors

H. El Yaakoubi; H. Boukhal; T. El Bardouni; L. Erradi; E. Chakir; K. Benaalilou; M. Lahdour; S. El Ouahdani; M. El Barbari

doi:10.1016/j.jksus.2019.02.004

View/Download PDF

Buy Reprints

PDF

Translate this page into:

31 (

); 1271-1275

doi:

10.1016/j.jksus.2019.02.004

Validation study of the reactor physics lattice transport code DRAGON5 & the Monte Carlo code OpenMC by critical experiments of light water reactors

H. El Yaakoubi^{^a,⁎}, H. Boukhal^{^a}, T. El Bardouni^{^a}, L. Erradi^{^b}, E. Chakir^{^c}, K. Benaalilou^{^a}, M. Lahdour^{^a}, S. El Ouahdani^{^a}, M. El Barbari^{^a}

a ERSN-LMR, University Abdelmalek Essaadi, Faculty of Sciences, Department of Physics, POB 2121, Tetouan, Morocco

b Faculty of Sciences, Rabat, Morocco

c SimoLab, Faculty of Sciences, University Ibn Tofail, Kenitra, Morocco

⁎Corresponding author. elyaakoubi.houda-etu@uae.ac.ma (H. El Yaakoubi)

Received: 2018-8-10, Accepted: 2019-2-4, Published: 2019-10-01

Disclaimer:
This article was originally published by Elsevier and was migrated to Scientific Scholar after the change of Publisher.

Peer review under responsibility of King Saud University.

Abstract

The aim of this study is to validate the reactor physics lattice transport code DRAGON5 and the Monte Carlo code OpenMC by neutronic analysis of critical experiments of light water cores. In this work the analysis of integral parameters of five light water reactor critical experiments TRX-1, TRX-2, BAPL-1, BAPL-2, and BAPL-3 is achieved based on evaluated nuclear data library ENDF/B-VII.1. BAPL and TRX experiments provide experimental buckling and are suitable benchmark lattices for validating the deterministic reactor physics lattice transport code DRAGON5 and the stochastic OpenMC code as well as evaluating nuclear data library. The integral parameters of the abovementioned critical experiments are calculated using DRAGON5 and OpenMC codes. To assess our calculation scheme the calculated integral parameters are compared to the measured values as well as the earlier published MCNP results based on the Chinese evaluated nuclear data library CENDL-3.0. Our calculations led to results in good agreement with the experiment and the earlier MCNP calculation results. Therefore, this study reveals the potential validation of the reactor physics lattice transport code DRAGON5 and the Monte Carlo code OpenMC using ENDF/B-VII.1 nuclear data library.

Keywords

TRX

BAPL

Critical experiments

Integral parameters

Code validation

DRAGON5

OpenMC

ENDF/B-VII.1

Show Related Articles from PubMed

1 Introduction

The light water reactors (LWR) such as the 2 MW TRIGA Mark-II research reactor are still an important component of modern reactor fuel management strategies. A lot of effort was devoted in the past to the development and to the validation of adequate neutron transport calculation codes for design calculation of LWRs, and various accurate neutronic calculations were performed using specific methods and nuclear data libraries. However, the frequent utilization of the existing code systems for calculations of LWR configurations requires a continuous and rigorous verification and validation of the results quality, especially when new nuclear data are used.

OpenMC (version 0.10) and DRAGON5 provide open-source implementations of stochastic and deterministic resolution techniques of neutron transport equation, respectively. Both codes are distributed by recognized North-American universities and are currently used worldwide.

The computer code DRAGON5 (Hébert, 2009; Marleau et al., 2017) is widely used for core calculations of a wide variety of thermal reactors. It consists of a transport code programmed by the Institute of Nuclear Engineering of Polytechnique Montréal, Canada. It is cell and assembly calculations software (Marleau et al., 2017) used to solve the neutron transport equation using the collision probability or characteristics methods. While the Monte Carlo code OpenMC (Romano et al., 2015) is a Monte Carlo particle transport simulation code focused on neutron calculations. It is capable of simulating 3D models based on constructive solid geometry with second-order surfaces.

Earlier studies performed by members of ERSN Laboratory of University Abdelmalek Essaadi (El Bakkari et al., 2010, 2013; Chham et al., 2016 ) have been focused on Monte Carlo modeling of The Moroccan TRIGA MARK II reactor by use of MCNP code.

The aim of this study is to validate the reactor physics lattice transport code DRAGON5 and the Monte Carlo code OpenMC by TRX and BAPL critical experiments of light water reactors for future neutronics analysis of 2 MW TRIGA Mark-II research reactor of CENM, Rabat, Morocco.

2 Material and methods

In this study, the input files of TRX and BAPL benchmark lattices of LWRs are modeled with DRAGON5 and OpenMC codes. The experiments analyzed in this paper are water-moderated lattices of slightly enriched (1.3 wt%) uranium metal rods (TRX-1 and TRX-2) and water-moderated critical lattices of 1.311 wt% enriched uranium oxide rods (BAPL-1 through BAPL-3). The calculations of integral parameters for TRX and BAPL benchmark lattices were carried out using the evaluated nuclear data libraries ENDF/B-VII.1. The calculated integral parameters were compared with the experimental and MCNP results based on CENDL-3.0 library (Hongwei, 2007).

2.1

2.1 Codes description

Neutronic calculations are based on “Deterministic” and “Stochastic” methods. In deterministic methods the transport equation is solved as a differential equation (Hébert, 2009). In stochastic methods such as Monte Carlo discrete particle histories are tracked and averaged in a random walk directed by interaction probabilities. Deterministic methods usually involve multi-group approaches while Monte Carlo can work with continuous energy cross-section libraries.

In this study, the reactor physics lattice transport code DRAGON5 and the Monte Carlo OpenMC code were used in our validation study through TRX and BAPL critical experiments of light water reactors.

2.1.1

2.1.1 DRAGON5

The computer code DRAGON5 (Marleau et al., 2017; Jaï et al., 2017) is an open-source code performed by the Institute of Nuclear Engineering of Polytechnique Montréal, Canada. The lattice DRAGON code is designed as a collection of modules that can simulate the neutron behavior of a unit cell or a fuel assembly in a nuclear reactor. It includes all of the functionalities that characterize a lattice cell code, namely: the interpolation of microscopic cross sections supplied by means of standard libraries; the resonance self-shielding calculations in multidimensional geometries; the multigroup and multidimensional neutron flux calculations that can take into account neutron leakage; the transport-transport or transport-diffusion equivalence calculations as well as editing of condensed and homogenized nuclear properties for reactor calculations; and finally, the isotopic depletion calculations.

2.1.2

2.1.2 OpenMC

OpenMC is a Monte Carlo particle transport simulation code (Romano et al., 2015) focused on neutron criticality calculations. It is capable of simulating 3D models based on constructive solid geometry with second-order surfaces. OpenMC supports either continuous-energy or multi-group transport. The continuous-energy particle interaction data is based on a native HDF5 format that can be generated from ACE files used by the MCNP and Serpent Monte Carlo codes. The actual nuclear data library adopted by OpenMC code is based on ENDF/B-VII.1 and provided by NNDC in ACE format (NNDC, 2011).

OpenMC was originally developed by members of the Computational Reactor Physics Group at the Massachusetts Institute of Technology starting in 2011. In the present work, we used ERSN-OpenMC, which is a Graphical User Interface for OpenMC (El Bakkali et al., 2014).

2.2

2.2 Description of benchmark experiments TRX and BAPL

In this paper, two thermal reactor benchmark cases, TRX and BAPL, from the Cross Section Working Group Benchmark Specifications (CSEWG) were used (CSEWG, 1986).

TRX experiments consist of two H₂O moderated lattices TRX-1 and TRX-2 of slightly enriched (Hardy, 1970) (1.3 wt%) uranium metal rods in a triangular pattern, with diameters of 0.98297 cm. Moreover, we treat the benchmark cores and Pin cell of TRX (Kobayashi and Zukeran, 1995; Okumura and Mori, 2003), cores which are consisting of 764 and 578 fuel rods for TRX-1 and TRX-2, respectively. A cross-section of a pin cell and full core of TRX is shown in Fig. 1.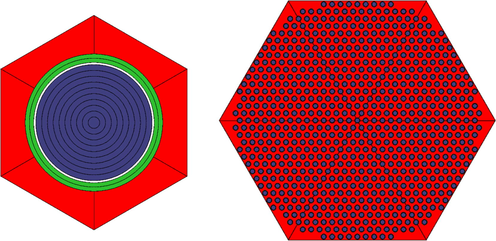

Cross-section of pin cell and the core with 764 rods of TRX-1 modeled by DRAGON5.

BAPL experiments are three H₂O moderated lattices BAPL-1, BAPL-2, and BAPL-3 of slightly enriched (Hellens et al., 1964) (1.311 wt%) uranium oxide rods with diameters of 0.9728 cm in a triangular pattern. Measured lattice parameters include ρ²⁸, δ²⁵, δ²⁸, and C* (definitions are given in Section 3). These lattices directly test the U-235 resonance fission integral and thermal fission cross section. They also test U-238 shielded resonance capture and the thermal capture cross section. They are sensitive to the U-238 fast fission cross-section, U-238 inelastic scattering, and the U-235 fission spectrum.

2.3

2.3 Description of materials and geometry of TRX and BAPL benchmarks

Tables 1 and 2 give the useful material and geometry characteristics of TRX (Brown et al., 1958) and BAPL (Hellens et al., 1964), respectively.

Table 1 Materials and geometry summary of TRX (Brown et al., 1958).

Pitch (cm)	TRX-1: 1.8060, 764 rods (hexagonal) TRX-2: 2.1740, 578 rods (hexagonal)
Water/fuel volume ratio	TRX-1: 2.35 TRX-2: 4.02
Moderator	H₂O
Fuel material	U (1.3 w% enrichment)
Fuel Isotopic Concentration (10²⁴ Atoms/cm³)	U-235: 0.0006253 U-238: 0.047205
Radius of fuel rods (cm)	0.4915
Clad material	Al
Clad Isotopic Concentration (10²⁴ Atoms/cm³)	Al: 0.06025
Outer radius of clad (cm)	0.5753
Thickness of clad (cm)	0.0711
Temperature (all components) (K)	293.0
Experimental buckling B² (cm⁻²)	TRX-1: 0.0057 ± 0.0001 TRX-2: 0.005469 ± 0.000036

Table 2 Materials and geometry summary of BAPL (Hellens et al., 1964).

Pitch (cm)	BAPL-1: 1.5578 (hexagonal) BAPL-2: 1.6523 (hexagonal) BAPL-3: 1.8057 (hexagonal)
Water/fuel volume.ratio	BAPL-1: 1.43 BAPL-2: 1.78 BAPL-3: 2.40
Moderator	H₂O
Fuel material	UO₂ (1.311 w% enrichment)
Fuel Isotopic Concentration (10²⁴ Atoms/cm³)	O: 0.046946 U-235: 0.0003112 U-238: 0.023127
Radius of fuel rods (cm)	0.4864
Clad material	Al
Clad Isotopic Concentration (10²⁴ Atoms/cm³)	Al: 0.06025
Outer radius of clad (cm)	0.5753
Thickness of clad (cm)	0.0711
Temperature (all components) (K)	293.0
Experimental buckling B² (cm⁻²)	BAPL-1: 0.003259 ± 0.000015 BAPL-2: 0.003347 ± 0.000015 BAPL-3: 0.003422 ± 0.000013

3 Integral parameters calculations

Results of original works on TRX and BAPL lattices are provided with experimental buckling values. These values are needed in DRAGON5 computing code to perform leakage calculation and model the finite medium. The OpenMC (version 0.10) Monte Carlo code is not designed to handle buckling during simulation. In this work we have used the experimental buckling values of TRX benchmarks to deduce the effective multiplication factors k_eff from infinite cell calculations. The absorption, fission, and total capture reaction rates for U-235 and U-238 isotopes are calculated at room temperature of 293 K, in the thermal and epithermal energy ranges using the codes DRAGON5 and OpenMC. The thermal cutoff energy in our calculations is set to 0.625 eV. For both codes neutron cross sections are processed based on the ENDF/B-VII.1 evaluated data file.

The integral parameters analyzed in this study are given by the following equations (Sher and Fiarman, 1976) are the effective multiplication factor and the spectral indices:

k_eff = finite medium effective multiplication factor
ρ²⁸ = Ratio of epithermal to thermal ²³⁸U captures
= (Σ_c)_epth³⁸/(Σ_c)_th³⁸ = (Σ_a − Σ_f)_epth³⁸/(Σ_a − Σ_f)_th³⁸
δ²⁵ = Ratio of epithermal to thermal ²³⁵U fission
= (Σ_f)_epth³⁵/(Σ_f)_th³⁵
δ²⁸ = Ratio of ²³⁸U fission to ²³⁵U fission
= (Σ^t_f)³⁸/(Σ^t_f)³⁵
C* = Ratio of ²³⁸U captures to ²³⁵U fission
= (Σ^t_c)³⁸/(Σ^t_f)³⁵
= (Σ^t_a-Σ^t_f)³⁸/(Σ^t_f)³⁵

3.1

3.1 DRAGON5 calculations

The TRX and BAPL benchmark lattices were modeled by DRAGON5 code explicitly in two-dimensional hexagonal geometry with reflective boundary condition. The maximum details of benchmark specifications are taken into account. The neutron transport equation resolution is performed in 2D geometry using the collision probability method (CP) (Hébert, 2009; Marleau et al., 2017) and invoking the self-shielding model implemented in DRAGON5 code. Various advanced self-shielding models are provided by the code. They are based on two main approaches: equivalence in dilution or subgroup models. In this study we restricted our calculations to use of self-shielding calculations based on equivalence in dilution model provided by SHI module of DRAGON5 code. Finally, as TRX and BAPL benchmarks are providing experimental buckling values, it is suitable to run calculations using a fixed buckling with k-infinite as the eigenvalue (type K). The resulting flux obtained using the 172 energy-groups library based on ENDF/B-VII.1 is used to calculate reaction rates defining the above spectral indices.

3.2

3.2 OpenMC calculations

Monte Carlo calculation of neutron flux and integral parameters is performed by means of our 3D model of the TRX and BAPL lattices. The OpenMC models are detailed as much as possible to minimize the effect of geometry and materials data uncertainties. To provide effective multiplication factors with statistical errors around 2 pcm, calculations were conducted in eigenvalue mode simulating a set of 2300 batches; in each batch 1,000,000 neutrons are tracked. In order to ensure the fission source convergence, we discarded 300 batches. Hence, all the reactions rates are obtained with relative statistical errors in the range 0.003%–0.005%. Continuous energy neutron data library based on ENDF/B-VII.1 were used in all Monte Carlo calculations.

4 Results and discussions

The integral parameters for TRX and BAPL thermal benchmark lattices are calculated using DRAGON5 and OpenMC codes. The obtained results are summarized in Tables 3–5 . For each benchmark lattice, the measured (CSEWG, 1986) and MCNP (Hongwei, 2007) calculated values as well as our calculated integral parameters are given. The associated relative deviation of calculated values from experiment are also provided.

Table 3 Integral parameters calculated by DRAGON5 and OpenMC for TRX pin cell.

Lattice	Integral parameter	Experimental^$ (CSEWG) (CSEWG, 1986)	MCNP^& (CENDL-3.0) (Hongwei, 2007)	DRAGON5^& (ENDF-B/VII.1)	OpenMC^& (ENDF-B/VII.1)
TRX-1	K_eff	1.0000(0.30)	0.9975(−0.25)	0.9988(−0.12)	1.0024(0.24)
	ρ²⁸	1.3200(1.60)	1.3608(3.09)	1.3600(3.03)	1.2900(−1.53)
	δ²⁵	0.0987(1.00)	0.0980(−0.71)	0.0954(−3.34)	0.0945(−4.25)
	δ²⁸	0.0946(4.30)	0.0962(1.69)	0.0965(2.01)	0.0893(−5.49)
	C*	0.7970(1.00)	0.7922(−0.60)	0.7930(−0.50)	0.7740(−2.84)

TRX-2	K_eff	1.0000(0.10)	0.9982(−0.18)	0.9991(−0.09)	1.0019(0.19)
	ρ²⁸	0.8370(1.90)	0.8530(1.91)	0.8700(3.94)	0.8150(−2.58)
	δ²⁵	0.0614(1.30)	0.0620(0.98)	0.0590(−3.90)	0.0582(−5.21)
	δ²⁸	0.0693(5.10)	0.0681(−1.73)	0.0709(2.30)	0.0654(5.98)
	C*	0.6470(0.93)	0.6387(−1.28)	0.6470(0.00)	0.6270(3.08)

$ Values in brackets are relative measurement uncertainties in %.

& The relative difference from measurement = [(calculated − experimental)/experimental] * 100 in %.

Table 4 Integral parameters calculated by DRAGON5 and OpenMC for TRX full core.

Lattice	Integral parameter	Experimental^$ (CSEWG) (CSEWG, 1986)	MCNP^& (CENDL-3.0) (Hongwei, 2007)	DRAGON5^& (ENDF-B/VII.1)	OpenMC^& (ENDF-B/VII.1)
TRX-1	K_eff	1.0000(0.30)	0.9975(−0.25)	0.9991(−0.09)	0.9981(−0.18)
	ρ²⁸	1.3200(1.60)	1.3608(3.09)	1.3540(2.57)	1.2600(−4.10)
	δ²⁵	0.0987(1.00)	0.0980(−0.71)	0.0968(−1.92)	0.0910(−7.80)
	δ²⁸	0.0946(4.30)	0.0962(1.69)	0.0921(−2.64)	0.0935(−1.16)
	C*	0.7970(1.00)	0.7922(−0.60)	0.8000(0.37)	0.7670(3.75)

TRX-2	K_eff	1.000(0.10)	0.9982(−0.18)	1.0004(0.04)	0.9979(0.20)
	ρ²⁸	0.8370(1.90)	0.8530(1.91)	0.8310(−0.71)	0.8010(−4.27)
	δ²⁵	0.0614(1.30)	0.0620(0.98)	0.0591(−3.74)	0.0569(−7.26)
	δ²⁸	0.0693(5.10)	0.0681(−1.73)	0.0706(1.87)	0.0683(−1.30)
	C*	0.6470(0.93)	0.6387(−1.28)	0.6510(0.61)	0.6220(−3.78)

$ Values in brackets are relative measurement uncertainties in %.

& In brackets: the relative difference from measurement = [(calculated − experimental)/experimental] * 100 in %.

Table 5 Integral parameters calculated by DRAGON5 and OpenMC for BAPL.

Lattice	Integral parameter	Experimental^$ (CSEWG) (CSEWG, 1986)	MCNP^& (CENDL-3.0) (Hongwei, 2007)	DRAGON5^& (ENDF-B/VII.1)	OpenMC^& (ENDF-B/VII.1)
BAPL-1	K_eff	1.0000(0.10)	1.0023(0.23)	0.9953(−0.47)	1.0017(0.17)
	ρ²⁸	1.3900(0.72)	1.3923(0.16)	1.4600(5.03)	1.3660(−1.68)
	δ²⁵	0.0840(2.40)	0.0820(−2.39)	0.0820(−2.38)	0.0799(−4.78)
	δ²⁸	0.0780(5.10)	0.0736(−5.61)	0.0769(−1.41)	0.0707(−9.36)
	C*	–	0.7972	0.8190	0.7890

BAPL-2	K_eff	1.0000(0.10)	1.0021(0.21)	1.0040(0.40)	1.0012(0.12)
	ρ²⁸	1.1200(0.89)	1.1602(3.59)	1.1900(6.25)	1.1380(1.64)
	δ²⁵	0.0680(1.50)	0.0669(−1.61)	0.0678(−0.29)	0.0652(−4.16)
	δ²⁸	0.0700(5.70)	0.0633(−9.57)	0.0704(0.57)	0.0635(−9.27)
	C*	–	0.7274	0.6500	0.7210

BAPL-3	K_eff	1.0000(0.10)	1.0021(0.21)	1.0010(0.10)	1.0004(0.04)
	ρ²⁸	0.9060(1.10)	0.9130(0.77)	0.9320(2.86)	0.8930(−1.36)
	δ²⁵	0.0520(1.90)	0.0515(−0.96)	0.0511(−1.73)	0.0508(−2.30)
	δ²⁸	0.0570(5.30)	0.0518(−9.12)	0.0548(−3.85)	0.0520(−8.76)
	C*	–	0.6511	0.6580	0.6480

$ Values in brackets are relative measurement uncertainties in %.

& The relative difference from measurement = [(calculated − experimental)/experimental] * 100 in %.

In comparison to the experiment results it is found that the calculated effective multiplication factors k_eff for TRX and BAPL lattices using the ENDF-B/VII.1 data library are generally in good agreement with experimental values. Nevertheless, the maximum uncertainty in k_eff was 0.47% for BAPL-1 obtained by DRAGON5 and 0.2% for TRX2 obtained by OpenMC in the case of full core simulation. In most TRX cases, pin cell and core calculated values of the remaining integral parameters show feeble errors compared to the measured values. These errors remain less than 4% for calculations achieved by DRAGON5 and 6% in the case of OpenMC calculations.

In the case of BAPL-2 and BAPL-3 OpenMC calculation slightly underestimates the values of δ²⁵ and δ²⁸ when compared to experiment and DRAGON5 calculated values. Nevertheless, similar behavior of these two parameters is seen in values calculated using MCNP (Hongwei, 2007). Since the measurements of parameter C* are not available for BAPL lattices, the comparison was done with values obtained by MCNP code of reference (Hongwei, 2007). The relative differences to experiment in the results based on ENDF/B-VII.1 evaluated data library by DRAGON5 and OpenMC codes are relatively improved.

Most of the DRAGON5 calculated parameters are within the uncertainty interval of the experiment results; Monte Carlo simulations relative errors to experiment remain in some cases higher than experimental uncertainties. Generally the obtained results indicate that the calculation schemes based on DRAGON5 and OpenMC codes and ENDF/B-VII.1 data file are reliable for the neutronics analysis of thermal reactors. So, this work could be an important contribution to basing future more extensive neutronic calculations of light water reactors especially the analysis of the Moroccan TRIGA MARK-II reactor.

5 Conclusion

In the present analysis, the critical experiments TRX and BAPL were studied for validating the deterministic DRAGON5 and the stochastic OpenMC codes as well as the nuclear data library ENDF/B-VII.1. By comparing the calculated results with experiment as well as earlier published MCNP values, it was found that the results of integral parameters show no significant differences between DRAGON5 and OpenMC. This reflects that the benchmark models developed by the two codes were good in predicting the integral parameters of TRX and BAPL benchmark lattices of light water reactors. The slight difference between the calculated results is due to the different solution methods of each code with its associated nuclear data. Therefore, this analysis validates the lattice transport code DRAGON5 and Monte Carlo code OpenMC, and it will be reliable to develop calculation scheme and nuclear data for further neutronic analysis of the 2 MW TRIGA MARK-II research reactor of Morocco.

References

Brown J.R., Harris D.R., Frantz F.S., Volpe J.J., Andrews J.C., Noordhoff B.H., . Kinetic and Buckling Measurements on Lattices of Slightly Enriched Uranium and UO₂ Rods in light water, WAPD-176. 1958.
Chham E., El Bardouni T., Benaalilou K., Boukhal H., El Bakkari B., Boulaich Y., El Younoussi C., Nacir B., . Neutronic and thermal–hydraulic analysis of new irradiation channels inside the Moroccan TRIGA Mark II research reactor core. Appl. Radiat. Isotop.. 2016;116(2016):178-184.
[Google Scholar]
CSEWG, . Cross Section Evaluation Working Group (CSEWG), Benchmark Specifications with Supplements. Upton, New York 11973, BNL-19302, II. ENDF-202, USA: Brookhaven National Laboratory, National Nuclear Data Center; 1986.
El Bakkali J., El Bardouni T., Safavi S.M., Boukhal H., Kaddour M., Benaalilou K., Chham E., . ERSN-OpenMC, a Java-based GUI for OpenMC Monte Carlo code. J. Radiat. Res. Appl. Sci.. 2014;9(3):234-241. ERSN-OpenMC software Available in
[Google Scholar]
El Bakkari B., Nacir B., El Bardouni T., El Younoussi C., Merroun O., Htet A., Boulaich Y., Zoubair M., Boukhal H., Chakir M., . Monte Carlo modelling of TRIGA research reactor. Radiat. Phys. Chem.. 2010;79(10):1022-1030.
[Google Scholar]
El Bakkari B., El Bardouni T., Nacir B., El Younoussi C., Boulaich Y., Boukhal H., Zoubair M., . Fuel burnup analysis for the Moroccan TRIGA research reactor. Ann. Nucl. Energy. 2013;51:112-119.
[Google Scholar]
Hardy J., . A Study of Physics Parameters in Several Water Moderated Lattices of Slightly Enriched and Natural Uranium, WAPD-TM-931. 1970.
Hébert A., . Applied Reactor Physics. Montréal: Presses Internationales Polytechnique; 2009. p. :424. ISBN 978-2-553-01436-9
Hellens R.L., . Reactor Physics Data for Water-Moderated Lattices of Slightly Enriched Uranium. Reactor Technology Selected Reviews 529, Price, G.A.. 1964.
Hongwei Y.U., . Present Status of CENDL Project. China Nuclear Data Center, China Institute of Atomic Energy; 2007. (April)
Jaï O., El Hajjaji O., Hébert A., . Validation study of the reactor physics lattice transport code DRAGON5 based on DRAGLIB libraries by TRX and BAPL critical experiments of light water reactors for neutronic analysis of TRIGA MARK-II research reactor. Moscow Univ. Phys.. 2017;72:633.
[CrossRef] [Google Scholar]
Kobayashi K., Zukeran A., . Three-dimensional benchmark tests of ENDF/B-VI for thermal reactor core. J. Nucl. Sci. Technol.. 1995;32(5):456-463.
[Google Scholar]
Marleau G., Hébert A., Roy R., . A User Guide for Dragon Version 5. Institut de génie nucléaire, École Polytechnique de Montréal; 2017.
NNDC, . ENDF/B-VII.1 Evaluated Nuclear Data Library. 2011.
[Google Scholar]
Okumura K., Mori T., . Integral Test of JENDL-3.3 for Thermal Reactors, JAERI CONF, JP0350312. 2003.
Romano P.K., Horelik N.E., Herman B.R., . OpenMC: a state-of-the-art, Monte Carlo code for research and development. Ann. Nucl. Energy. 2015;82:90-97.
[CrossRef] [Google Scholar]
Sher R., Fiarman S., . Studies of Thermal Reactor Benchmark Data Interpretation: Experimental Corrections. EPRI NP-209. 1976.