Translate this page into:
On the characteristics of head on collision of dust ion acoustic solitons in the adiabatic dusty plasmas
*Tel.: +967 771848483 mahkhaled@hotmail.com (M.A.H. Khaled)
-
Received: ,
Accepted: ,
This article was originally published by Elsevier and was migrated to Scientific Scholar after the change of Publisher.
Available online 21 April 2014
Peer review under responsibility of King Saud University.

Abstract
Based on a one-dimensional mode, the characteristics of the head on collision between two dust ion acoustic solitons (DIASs), propagating in opposite directions in adiabatic dusty plasmas composed of adiabatic non-inertial electrons, adiabatic inertial ions and immersed (negatively/positively) charged dust grains have been investigated. The extended Poincaré–Lighthill–Kuo (PLK) method has been used to obtain two side Korteweg-de Vries (KdV) equations for DIASs. The analytical phase shifts and trajectories after collision of two solitons are given. The effects of adiabaticity of electron and ion fluids, concentration of negatively/positively charged static dust particles and ion temperature to electron temperature ratio on the phase shift are studied. It is found that these factors significantly affect the phase shifts.
Keywords
Adiabatic dusty plasma
Dust ion acoustic solitons
PLK method
KdV equations
Phase shift
1 Introduction
Study of various types of nonlinear phenomena in dusty plasmas is one of the most important topics in the modern plasma physics researches because of their relevance in astrophysical and space environments such as comettails, planetary ring, asteroid zones, interstellar medium, lower part of Earth’s ionosphere and magnetosphere radio frequency plasma discharge (Horányi and Mendis, 1985; Northrop, 1992; Mendis, and Rosenberg, 1994; Verheest, 2000; Shukla and Mamun, 2002). In dusty plasmas, due to the presence of a high density of dust grains, different types of collective processes exist and new wave modes can be exciting. One of these modes is the low-frequency dust ion acoustic waves (DIAWs), theoretically predicted by Shukla and Silin (1992) in an unmagnetized dusty plasma and then these waves have been observed in the laboratory experiments (Barkan et al., 1996). In most space and laboratory dusty plasma environments, dust grains, immersed in the plasma would be essentially charged by the capture of the more mobile electrons; hence, they become negatively charged (Shukla and Mamun, 2002). On the other hand, the existence of positively charged dust in different regions of space (Viz., upper part of ionosphere, lower part of magnetosphere, in the Earth’s mesosphere and cometary tail etc. as well as laboratory environments) was also observed (Horányi et al., 1993; Rosenberg and Mendis, 1995; Rosenberg et al., 1999; Samarian et al., 2001). Generally, there are a number of principle mechanisms by which a dust grain becomes positively charged. These include secondary emission of electrons from the surface of the dust grains, photo electron emission by UV radiation, thermionic emission, field emission, impact ionization, etc., (Rosenberg and Mendis, 1995; Rosenberg et al., 1999). There is also direct evidence for the existence of both positively and negatively charged dust particles in space and laboratory plasmas (Zhao et al., 2002; Mamun, 2008a). It is expected that the presence of such negative (positive) dust grain can modify or introduce new features in the propagation of the dust associated waves, particularly, the electrostatic waves of Shukla and Silin (1992). Different plasma modes arise due to a delicate balance between nonlinearity and dispersion, which leads to the generation of solitons. Currently, in the process of soliton propagation in dusty plasmas interaction represents one of the most interesting and important nonlinear phenomenon in modern plasma researches. A soliton [which was first remarked by Zabusky and Kruskal (1965)] is usually defined as a type of solitary waves which maintains its identity after its colliding with another soliton of the same kind. In fact, the solitons and their interactions have received a special attention due to the realization of their occurrence in most of different dusty plasma models. In a one dimensional model, there are two different methods to soliton interactions. One is overtaking collision which is studied by inverse scattering transformation method (Gardner et al., 1967) and the other is a head-on collision of two solitons traveling in the opposite directions (i.e., the angle between two propagation directions of two solitons is ) (Su and Mirie, 1980). Generally, for the problem of the head-on collision between two solitons, it is necessary to use or employ a suitable asymptotic expansion to solve original fluid dynamic equations in order to give the interesting features of the trajectories of solitons after collision and the corresponding phase shift. Many authors investigated the head-on collision of two solitary waves in many plasma models (Han et al., 2008; El-Shamy et al., 2009; El-Labany et al., 2010; El-Shamy, 2010; Chatterjee, 2010; Chatterjee and Ghosh, 2011; Ghosh et al., 2012; El-Shamy and Awad, 2012; Ghorui et al., 2013) by using extended Poincaré–Lighthill–Kuo (PLK) method (Jeffrey and Kawahara, 1982). For example, Han et al. (2008) studied the combined effects of electron to positron temperature ratio and the ratio of the number density of positrons to that of electrons on the phase shifts during the head-on collision between two IASWs. They found out that the ratio of electron temperature to positron temperature, and the ratio of the number density of positrons to that of electrons have significant influence on the phase shift. El Shamy (2010) studied the head-on collisions of ion thermal solitary waves in pair-ion plasma containing positive ions, negative ions and stationary (positively/negatively) charged dust grains. They found out that the phase shift is significantly affected by the presence of the positive-to-negative ion temperature ratio and positively/negatively charged dust grains. Chatterjee et al. (2010) investigated the head-on collision of ion acoustic solitary waves in electron-positron-ion plasma with superthermal electrons and Maxwell distribution of positrons. Chatterjee and Ghosh (2011) studied the head-on collision of IASWs in electron-positron-ion plasma. They took the non-Maxell’ distribution (superthermal) of both electrons and positrons. They illustrated that the ratio of electron temperature to positron temperature and the ratio of the number density of positrons to that of electrons had significant influence on the phase shift of the soliton. Ghosh et al. (2012) studied the head-on collision of IASWs in two component unmagnetized plasma with cold ions and nonextensive distributed electrons. They found that the presence of nonextensive distributed electrons played a significant role in the nature of collision of IASWs. Recently, Ghorui et al. (2013) investigated the head on collision between two DIASWs in magnetized quantum dusty plasma with positively/negatively charged dust grains. They observed that the phase shifts were significantly affected by the quantum diffraction parameter, the ion cyclotron frequency and the ratio of the densities of electrons to ions. Very recently, Khaled (2014) studied the head on collision between two IASWs in a weakly relativistic plasma containing nonextensive electrons and positrons. He found that the effects of the nonextensive parameter, positron-to-electron density ratio, ion-to-electron temperature ratio, electron-to-positron temperature ratio and relativistic factor had significant influence on the phase shift.
To the best of our knowledge, the head-on collision of two solitons in dusty plasma containing non-inertial adiabatic electron fluid, inertial adiabatic ion fluid and negatively/positively charged static dust particles have not yet been studied. Therefore, this paper aims to study the same topic mentioned above by using the extended PLK method. It also attempts to study the effects of the adiabaticity of electrons and ions, ion-to-electron temperature ratio and the concentration of negatively/positively charged dust particles on the characteristics of head-on collisions of solitons.
The manuscript is organized as follows. The governing equations of one-dimensional DIASs are shown in Section 2. In Section 3, the KdV equations are derived. Also the analytical phase shifts and trajectories from the original basic equations are obtained in Section 3. Results and discussion are given in Section 4. The conclusions are finally provided in Section 5.
2 Basic equations
We consider an unmagnetized adiabatic dusty plasma system composed of hot adiabatic inertial ion fluid, hot adiabatic non-inertial electrons fluid and stationary positively/negatively charged dust grains. Thus, at equilibrium, we have
where
(
), is the equilibrium number density of ions (electrons),
is the number density of static dust grain and
is the equilibrium number of charges residing on the dust grain surface.
for negative (positive) dust grains. Also, we consider the time scale of DIASs is much faster than the dust plasma period, so that the dust grains can be considered as stationary. Accordingly, the nonlinear dynamics of DIASs is described by (Mamun, 2008b).
Here Eq. (1) represents the normalized continuity equation for particle species j (with for electron and for ion), Eq. (2) represents the normalized momentum equation for adiabatic inertial ion fluid and Eq. (3) represents the normalized pressure equation for adiabatic particle species where . Eq. (4) represents the normalized momentum equation for non-inertial electron and Eq. (5) is normalized Poisson equation. In the above equations, is the particle number density of the particle specie j (where j = e,i) normalized by its equilibrium value . is the fluid thermal pressure of particle specie , normalized by where is the equilibrium temperature of the species in the energy unit. is the fluid velocity of particle species normalized by dust ion acoustic speed , with being the mass of ion. is the adiabatic index (i.e. heat capacity ratio) and is the electrostatic potential normalized by with being the magnitude of the electron charge. The time ( ) and space ( ) variables are normalized by the inverse of plasma frequency and the Debye length , respectively. We have defined , and .
3 Derivation of KdV equations and phase shifts
We assume that there are two solitons
and SB in our adiabatic dusty plasma system, which are asymptotically far apart in the initial states and travel toward each other. After some time they interact and collide with each other and then depart. We also assume that, the solitons have small amplitudes ∼
(where
is a smallness formal perturbation parameter characterizing the strength of nonlinearity). The interactions between two solitons are assumed to be weak. Hence, we expect that the collision will be quasielastic. In order to analyze the effects of collision, we employ an extended PLK perturbation method. According to this method, we introduce the stretched coordinates as
Introducing expansions (6–9) into Eqs. (1)–(5) and by equating the powers of
, we finally obtain the lowest order differential equations:
Solving the system of Eqs. (10)–(15), we finally obtain the following relations among different physical quantities:
From the condition that a unique solution of Eqs. (10)–(16) can be obtained when
is given by Eq. (22), the wave velocity
is also obtained. The unknown functions
and
will be determined from the next orders. Eqs. (17)–(22) imply that at the leading order, we have two waves, one of which,
, is traveling to right and the other one,
is traveling to left. At the next order, we have a system of equations whose solutions are given by
To the next higher order, we can deduce
Integrating Eq. (29) with respect to the variables
and
, we have
The third and fourth terms in Eq. (30) are not secular terms in this order, but they will become secular in the next order. Hence, we have:
Eqs. (31) and (32) represent the two side traveling wave KdV equations in the reference frames of
and
, respectively. The KdV equations given by Eqs. (31) and (32) have the following soliton solutions:
Therefore, up to
the trajectories of the two solitons for weak head-on interactions are
Now, to obtain the phase shifts after the interaction between two solitons, we shall assume that, the solitons characterized by
and SB are asymptotically far from each other at the initial time
, soliton
is at
,
and the soliton
is at
,
, respectively. After the collision
, the soliton
is far to the right of soliton
, i.e., the soliton
is at
,
and the soliton
is at
,
. Using Eqs. (39) and (40) we obtain the corresponding phase shift
and
as follows
4 Results and discussion
In this paper, we have studied the head-on collision of two DIASs in collisionless unmagnetized adiabatic dusty plasma comprising inertialless adiabatic electrons fluid, inertial adiabatic ions fluid, and (positively/negatively) charged stationary dust grains by using the extended PLK perturbation method. We have derived the KdV equations and analytical phase shifts of the head-on collision between two DIASs. Before going into details, it should be mentioned here that in a nonlinear dispersive media, solitary waves are formed due to the balance between the nonlinearity and dispersion. The coefficient of nonlinearity A and the coefficient of dispersion B, which appear in the soliton like solutions represented by Eqs. (31) and (32), may create two possibilities namely and . When , Eqs. (35) and (36) give the compressive solutions with and . On the other hand, when , Eqs. (35) and (36) give the rarefactive solutions with and . Also, it is worth to notice that each DIAS has a positive (negative) phase shift in its traveling direction. The positive or negative phase shift does not depend on the type of mode (i.e., ion-acoustic, dust-ion-acoustic, dust-acoustic, and electrostatic waves). It is well known that whether the phase shift is positive or negative it depends on the coefficient D, which appears in Eqs. (33) and (34). The positive (negative) phase shift after interaction between two solitons means that the velocities of the propagated solitons are averagely slower (faster) than the solitons without interactions. Eqs. (41) and (42) show that the magnitude of the phase shifts is directly related to the physical parameters i.e., and .
The effects of adiabaticity electrons and ions ( ), the concentration of positively/negatively charged dust grains ( ) and the ion-to-electron temperature ratio ( ) on the phase shifts after collision between two solitons are studied as follows.
Since soliton
is traveling to the right and soliton
is traveling to the left, Eqs. (41) and (42) show that, due to the collision, each soliton has a phase shift in its traveling direction. Here, all physical quantities are normalized and
and
(El-Shamy, 2010; Han et al 2008) are used. In order to know how these parameters affect on the phase shift, the results are given in Figs. 1–4. Fig. 1 (Fig. 2) shows the variation of the phase shift
with the concentration of negatively (positively) charged dust grains
for different values of ion-to-electron temperature ratio
in the adiabatic dusty plasma (i.e. when
). It is obvious from Fig. 1 that the phase shift
is always negative for any possible value of concentration of negatively charged dust grains
, and the negative value of phase shift
increases as
increases, but decreases as
increases. Fig. 2 indicates that, due to the presence of concentration of the positively charged dust grains in our adiabatic dusty plasma system, the value of phase shift
is always positive and increases as the positively charged dust grains
increases, but decreases as
increases. Moreover, From Fig. 2, it is found that, for small values of
(i.e., when
), the ion-to-electron temperature ratio
has a weak effect on the phase shift
, and for large values of
(i.e., when
)
has a strong effect on
. In addition, Fig. 2 shows that the phase shift does not change in the case of large values of
and
(i.e., when
and
).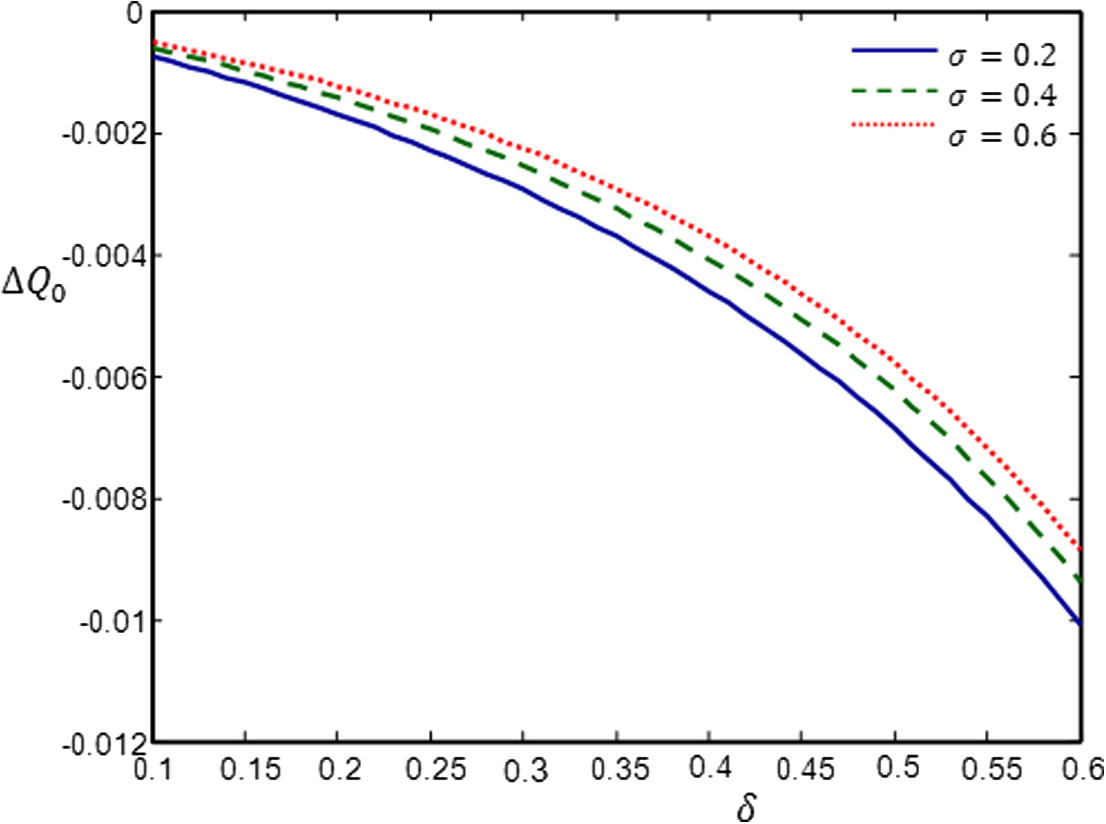
Variation of phase shift
with the concentration of negatively charged dust impurities
for different values of
and for
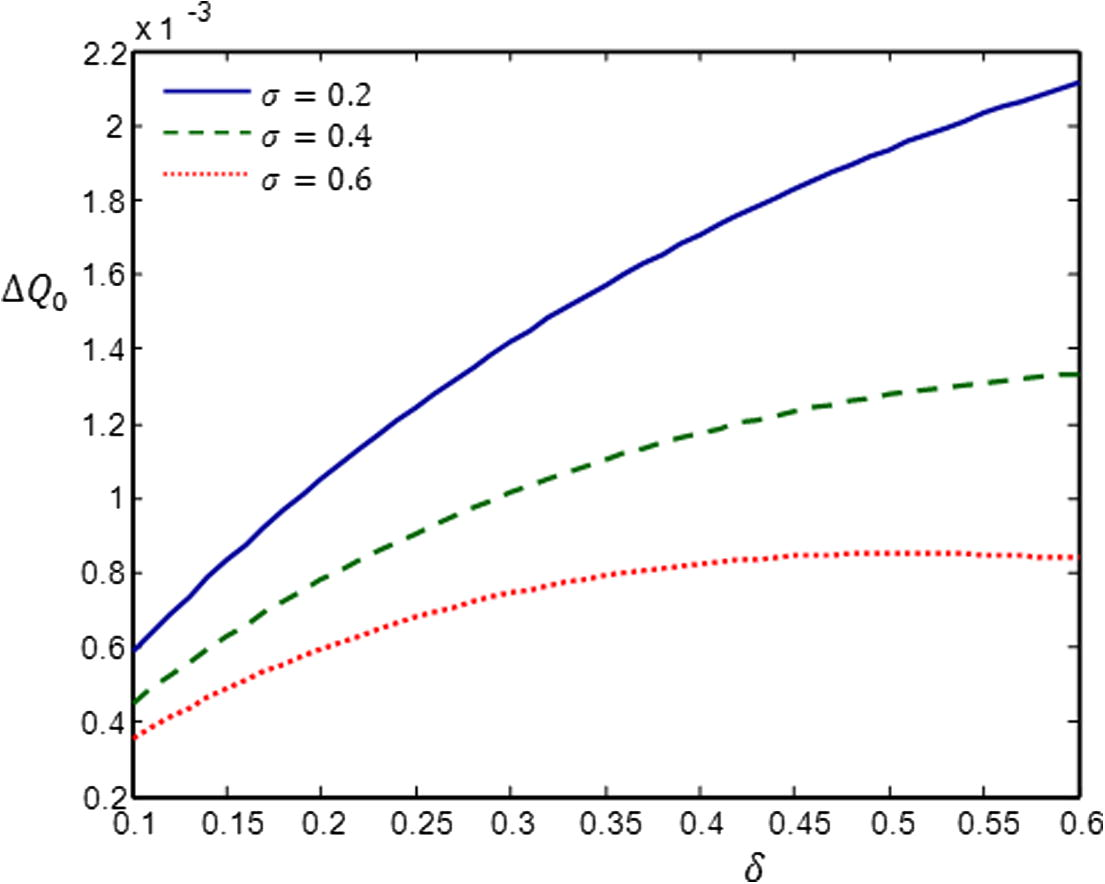
Variation of phase shift
with the concentration of positively charged dust impurities
for different values of
and for

Variation of phase shift
with the concentration of negatively charged dust impurities for
and
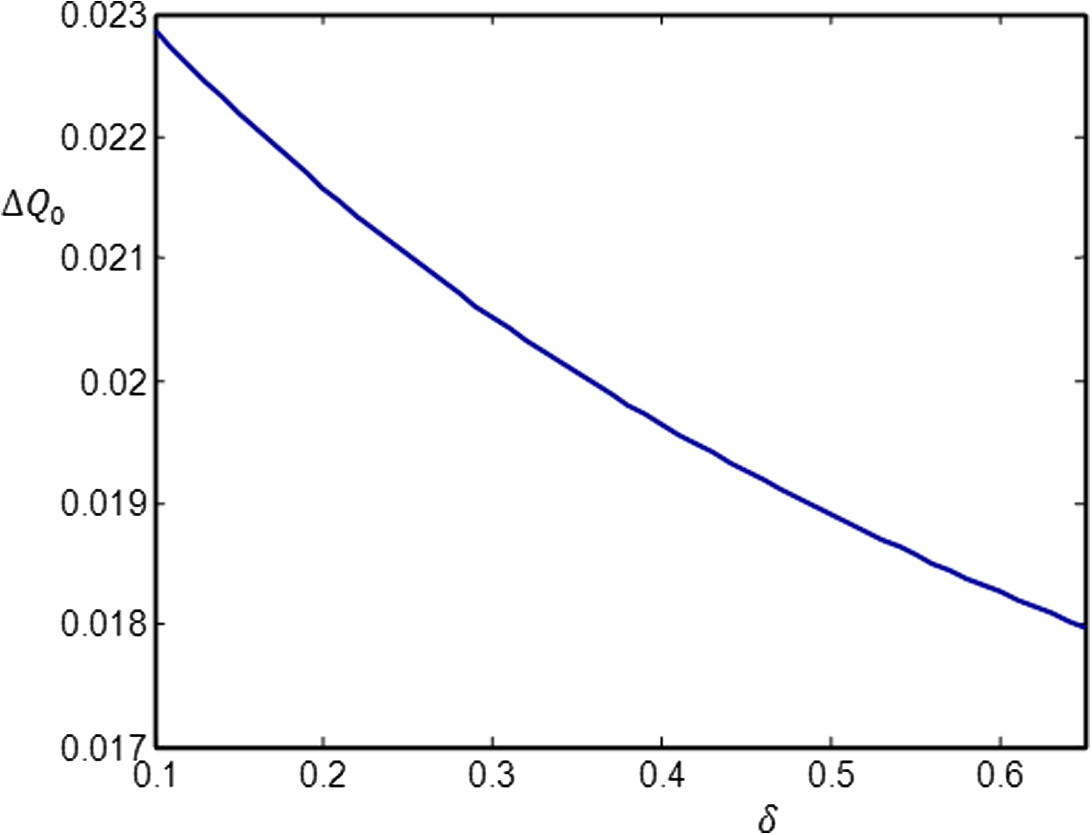
Variation of phase shift
with the concentration of positively charged dust impurities for
and
.
To compare, it is very important to mention here that for an isothermal dusty plasma i.e, when and , where is constant (i.e., ), Eqs. (1) and (3) are identical. It is also important to note that for isothermal electrons, Eq. (4) reduces to . Thus, the phase velocity of soliton is assumed to be much slower than the electron thermal velocity (i.e., isothermal electron) but much faster than the ion thermal velocity (i.e., cold ion). The case of cold ion and isothermal (Maxwellian) electrons is not valid for adiabatic inertial ions and adiabatic inertialless electrons. Fig. 3 demonstrates the variation in the phase shift with concentration of the negatively charged dust grains for cold ions (i.e. ) and isothermal electron (i.e. ). It is obvious from Fig. 3 that the magnitude of phase shift is always positive which is not found to be in adiabatic dusty plasma (Fig. 1) for any possible value of . Fig. 3 also indicates that the phase shift increases as increases. In addition, Fig. 3 illustrates that for the phase shift increases slowly and then it increases rapidly when . Fig. 4 represents the variation of with concentration of the positively charged dust grains for cold ions (i.e. ) and isothermal electron (i.e. ). It is clear that the magnitude of phase shift is decreasing with increase in the concentration of the positively charged dust grains which cannot be obtained in adiabatic dusty plasma (Fig. 2). Moreover, in the presence of positive dust component the magnitude of phase shift is larger compared to that of adiabatic dust plasma (Fig. 2). Therefore, we can conclude that the phase shift is modified significantly due to the effects of adiabaticity of electrons and ions.
5 Conclusions
In summary, the adiabatic electrons and ions, ion-to-electron temperature ratio and negatively/positively charged dust grains significantly modify the phase shift during interactions between two solitons. It has also been observed that, in the adiabatic dusty plasma containing negatively charged dust grains, there is no a positive phase shift (Fig. 1) [which are found to exist in dusty plasma containing isothermal electrons, cold ions for any possible value of negatively charged static dust grains (Fig. 3)]. On the other hand, in an adiabatic dusty plasma containing positively charged dust impurities, there is a positive phase shift (Fig. 2) [which are also found to exist in a dusty plasma containing isothermal electrons, cold ions and positively charged dust grains (Fig. 4)] for any possible set of dusty plasma parameters. Thus, the adiabatic index and negatively/positively charged static dust impurities are found to play an important role in the magnitude of phase shift of the DIASs. The ranges of different dusty plasma parameters used in this investigation are very wide ( and ), and are relevant to both space and laboratory dusty plasmas (Shukla and Mamun, 2002; Mamun, 2008; Mendis and Rosenberg, 1994; Barkan et al., 1996). Thus, the present investigation might be helpful to understand the collective phenomena related to DIASs collisions that are of vital importance in laboratory plasmas, space plasma as well as in plasma applications.
Acknowledgements
The author would like to thank the referees for a number of valuable criticisms and comments that have led to improvement of the original manuscript. The author also thanks the editor and his staff for their kind cooperation.
References
- Experiments on ion acoustic waves in dusty plasmas. Planet. Space Sci.. 1996;44:239.
- [Google Scholar]
- Head-on collision of ion acoustic solitary waves in electron-positron-ion plasma with superthermal electrons and positrons. Eur. Phys. J. D. 2011;64:413.
- [Google Scholar]
- Head-on collision of ion acoustic solitary waves in an electron–positron–ion plasma with superthermal electrons. Phys. Plasmas. 2010;17:122314.
- [Google Scholar]
- Head-on collision of dust-acoustic solitary waves in an adiabatic hot dusty plasma with external oblique magnetic field and two-temperature ions. Astrophys. Space Sci.. 2010;325:201.
- [Google Scholar]
- Head-on collision of ion thermal solitary waves in pair-ion plasmas containing charged dust impurities. Eur. Phys. J. D. 2010;56:73.
- [Google Scholar]
- On the characteristics of the head-on collision between two ion thermal waves in isothermal pair-ion plasmas containing charged dust grains. Chaos Solitons Fractals. 2012;45:15.
- [Google Scholar]
- Head-on collision of ion-acoustic solitary waves in a Thomas–Fermi plasma containing degenerate electrons and positrons. Phys. Lett. A. 2009;374:290.
- [Google Scholar]
- On the head-on collision between two ion acoustic solitary waves in a weakly relativistic plasma containing nonextensive electrons and positrons. Astrophys. Space Sci.. 2014;350:607.
- [Google Scholar]
- Head on collision of dust ion acoustic solitary waves in magnetized quantum dusty plasmas. Astrophys. Space Sci.. 2013;343:639.
- [Google Scholar]
- The effect of q-distributed electrons on the head-on collision of ion acoustic solitary waves. Phys. Plasmas. 2012;19:012113.
- [Google Scholar]
- Head-on collision of ion-acoustic solitary waves in a weakly relativistic electron–positron–ion plasma. Phys. Lett. A. 2008;372:4817.
- [Google Scholar]
- Trajectories of charged dust grains in the cometary environment. Astrophys. J.. 1985;294:357.
- [Google Scholar]
- Mechanism for the acceleration and ejection of dust grains from Jupiter’s magnetosphere. Nature. 1993;363:144.
- [Google Scholar]
- Asymptotic Methods in Nonlinear Wave Theory. London: Pitman; 1982.
- Electrostatic solitary structures in a dusty plasma with dust of opposite polarity. Phys. Rev. E. 2008;77:026406.
- [Google Scholar]
- Effects of adiabaticity of electrons and ions on dust-ion-acoustic solitary waves. Phys. Lett. A. 2008;372:1490.
- [Google Scholar]
- Conditions are investigated for forming a Coulomb lattice of dust grains which are charged positively by the ultraviolet induced photoemission of electrons. IEEE Trans. Plasma Sci.. 1995;23:177.
- [Google Scholar]
- Positively charged dust crystals induced by radiative heating. IEEE Trans. Plasma Sci.. 1999;27:239.
- [Google Scholar]
- Shukla, P.K., Mamun, A.A., 2002. Introduction to Dusty Plasma Physics, Institute of Physics Publishing, Bristol.
- Verheest, F., 2000. Waves in Dusty Space Plasmas. Kluwer Academic Publishers, Dordrecht.
- Interaction of solitons in a collisionless plasma and the recurrence of initial states. Phys. Rev. Lett.. 1965;15:240.
- [Google Scholar]
- The measurement of bipolar charge in poly-disperse powders using a vertical array of faraday pail sensors. J. Electrost.. 2002;55:261.
- [Google Scholar]







