Numerical solution of the linear two-dimensional Fredholm integral equations of the second kind via two-dimensional triangular orthogonal functions

Farshid Mirzaee; Sima Piroozfar

doi:10.1016/j.jksus.2010.04.007

View/Download PDF

Buy Reprints

PDF

Translate this page into:

ORIGINAL ARTICLE

22 (

); 185-193

doi:

10.1016/j.jksus.2010.04.007

Numerical solution of the linear two-dimensional Fredholm integral equations of the second kind via two-dimensional triangular orthogonal functions

Farshid Mirzaee^*, Sima Piroozfar

Department of Mathematics, Faculty of Science, Malayer University, Malayer 65719-95863, Iran

*Corresponding author. Tel./fax: +98 851 3339944 f.mirzaee@malayeru.ac.ir (Farshid Mirzaee) mirzaee@mail.iust.ac.ir (Farshid Mirzaee)

Received: 2010-3-27, Accepted: 2010-4-20, Published: 2010-10-01

Disclaimer:
This article was originally published by Elsevier and was migrated to Scientific Scholar after the change of Publisher.

Abstract

In this paper, we will give some results for developing the two-dimensional triangular orthogonal functions (2D-TFs) for numerical solution of the linear two-dimensional Fredholm integral equations of the second kind. The product of 2D-TFs and some formulas for calculating definite integral of them are derived and utilized to reduce the solution of two-dimensional Fredholm integral equation to the solution of algebraic equations. Also a theorem is proved for convergence analysis. Numerical examples are presented and results are compared with analytical solution to demonstrate the validity and applicability the method.

Keywords

Linear two-dimensional Fredholm integral equations of the second kind

Triangular orthogonal functions

Two-dimensional triangular orthogonal functions

Orthogonal functions

Show Related Articles from PubMed

1 Introduction

Many problems in engineering and mechanics can be transformed into two-dimensional Fredholm integral equations of the second kind. For example, it is usually required to solve Fredholm integral equations in the calculation of plasma physics (Farengo et al., 1983). There are many works on developing and analyzing numerical methods for solving Fredholm integral equations of the second kind (see Alipanah and Esmaeili, 2009; Alpert et al., 1993; Anselon, 1971; Atkinson, 1976, 1997; Baker, 1977; Delves and Mohamed, 1985 ). The subject of the presented paper is applying the 2D-TFs method for solving two-dimensional linear Fredholm integral equations. For this purpose we consider the two-dimensional Fredholm integral equations of the form

(1)

u_{1} (τ, μ) = f_{1} (τ, μ) + \int_{a}^{b} \int_{c}^{d} K_{1} (τ, μ, λ, η) u (λ, η) d λ d η; (τ, μ) \in D,

where

f_{1} (τ, μ)

and

K_{1} (τ, μ, λ, η)

are given continuous functions defined, respectively, on

D = L^{2} ([a, b] \times [c, d]), E = D \times D

and

u_{1} (τ, μ)

is unknown on D.

2 Review of two-dimensional block pulse functions (2D-BPFs)

As shown in Maleknejad et al. (2010), a set of 2D-BPFs $ϕ_{i, j} (x, y) (i = 0, 1, \dots, m_{1} - 1; j = 0, 1, \dots, m_{2} - 1)$ is defined in the region of $x \in [0, 1)$ and $y \in [0, 1)$ as:

(2)

ϕ_{i, j} (x, y) = \{\begin{matrix} 1, & {ih}_{1} ⩽ x < (i + 1) h_{1} and {jh}_{2} ⩽ y < (j + 1) h_{2}, \\ 0, & Otherwise, \end{matrix}

where

m_{1}, m_{2}

are arbitrary positive integers, and

h_{1} = \frac{1}{m_{1}}, h_{2} = \frac{1}{m_{2}}

According to (2), the interval $[0, 1)$ and $[0, 1)$ are, respectively, divided into $m_{1}$ and $m_{2}$ subintervals.

One of the important properties of the 2D-BPFs is the disjointness of them,

(3)

ϕ_{i_{1}, j_{1}} (x, y) ϕ_{i_{2}, j_{2}} (x, y) = \{\begin{matrix} ϕ_{i_{1}, j_{1}} (x, y), & i_{1} = i_{2} and j_{1} = j_{2}, \\ 0, & Otherwise, \end{matrix}

where

i_{1}, i_{2} = 0, 1, \dots, m_{1} - 1; j_{1}, j_{2} = 0, 1, \dots, m_{2} - 1

The orthogonality of 2D-BPFs is derived immediately from

(4)

\int_{0}^{1} \int_{0}^{1} ϕ_{i_{1}, j_{1}} (x, y) ϕ_{i_{2}, j_{2}} (x, y) dy dx = \{\begin{matrix} h_{1} h_{2}, & i_{1} = i_{2} and j_{1} = j_{2}, \\ 0, & Otherwise . \end{matrix}

The other property is completeness. For every

f (x, y) \in L^{2} ([0, 1) \times [0, 1))

when

m_{1}

and

m_{2}

approach to the infinity, Parseval's identity holds:

(5)

\int_{0}^{1} \int_{0}^{1} f^{2} (x, y) dy dx = \sum_{i = 0}^{\infty} \sum_{j = 0}^{\infty} f_{i, j}^{2} ‖ ϕ_{i, j} (x, y) ‖^{2},

where

(6)

f_{i, j} = \frac{1}{h_{1} h_{2}} \int_{0}^{1} \int_{0}^{1} f (x, y) ϕ_{i, j} (x, y) dy dx .

In vector form, an arbitrary function can be expanded in the form

f (x, y) = F^{T} ϕ (x, y) = ϕ^{T} (x, y) F

, where

F = [f_{0, 0}, \dots, f_{0, m_{2} - 1}, \dots, f_{m_{1} - 1, 0}, \dots, f_{m_{1} - 1, m_{2} - 1}]^{T},

with

f_{i, j}

's as defined in (6) (see Maleknejad et al., 2010).

3 Two-dimensional triangular orthogonal functions

We usually call the triangular orthogonal functions containing one variable as one-dimensional (1D) triangular orthogonal functions (1D-TFs) and those containing two variables as two-dimensional (2D) triangular orthogonal functions (2D-TFs). 1D-TFs have been widely used for solving different problems (Babolian et al., 2008; Deb et al., 2006). A complete detail for 1D-TFs is given in Babolian et al. (2008, 2006). These discussions can also be extended to the 2D-TFs.

3.1

3.1 Definition

In the following, we have dissected a 2D-BPFs into two 2D-TFs as shown in Fig. 1. Thus, we have

(7)

ϕ_{0, 0} (x, y) = T 1_{0, 0} (x, y) + T 2_{0, 0} (x, y) .

Now, we demonstrate the construction of 2D-TFs according to

(8)

ϕ_{i, j} (x, y) = T 1_{i, j} (x, y) + T 2_{i, j} (x, y),

where

T 1_{i, j} (x, y)

is defined as

(9)

T 1_{i, j} (x, y) = \{\begin{matrix} 1 - \frac{y - {jh}_{2}}{h_{2}}, & {ih}_{1} ⩽ x < (i + 1) h_{1} \\ and {jh}_{2} ⩽ y < (j + 1) h_{2}, \\ 0, & Otherwise, \end{matrix}

and

T 2_{i, j} (x, y)

is defined as

(10)

T 2_{i, j} (x, y) = \{\begin{matrix} \frac{y - {jh}_{2}}{h_{2}}, & {ih}_{1} ⩽ x < (i + 1) h_{1} and {jh}_{2} ⩽ y < (j + 1) h_{2}, \\ 0, & Otherwise, \end{matrix}

where

h_{1} = \frac{1}{m_{1}}, h_{2} = \frac{1}{m_{2}}

i = 0, 1, \dots, m_{1} - 1, j = 0, 1, \dots, m_{2} - 1

. Therefore, for each

ϕ_{i, j} (x, y), i = 0, 1, \dots, m_{1} - 1, j = 0, 1, \dots, m_{2} - 1

T 1_{i, j} (x, y)

and

T 2_{i, j} (x, y)

can be constructed as above.

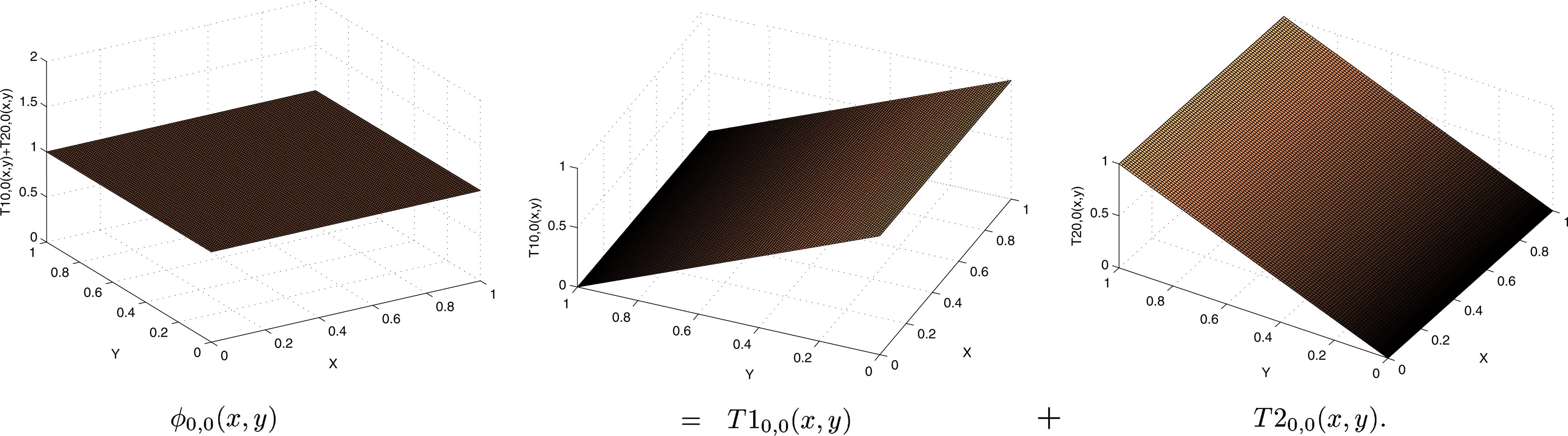
Dissection of ϕ 0 , 0 ( x , y ) into two 2D-TFs.

3.2

3.2 Vector forms

We can generate two vectors of orthogonal 2D-TFs, namely $T 1 (x, y)$ and $T 2 (x, y)$ , such that

(11)

ϕ (x, y) = T 1 (x, y) + T 2 (x, y); (x, y) \in [0, 1) \times [0, 1) .

It could be said that these two vectors are complementary to each other as far as 2D-BPFs are considered. We call T1(x, y) and T2(x, y) the left-handed two-dimensional triangular functions (LH2D-TFs) and the right-handed two-dimensional triangular functions (RH2D-TFs), respectively. Now, if we divide the interval

[0, 1) \times [0, 1)

m_{1} m_{2}

equal parts, we have

(12)

\begin{matrix} T 1 (x, y) = [T 1_{0, 0} (x, y), \dots, T 1_{0, m_{2} - 1} (x, y), \dots, T 1_{m_{1} - 1, 0} (x, y), \\ \dots, T 1_{m_{1} - 1, m_{2} - 1} (x, y)]^{T}, \end{matrix}

(13)

\begin{matrix} T 2 (x, y) = [T 2_{0, 0} (x, y), \dots, T 2_{0, m_{2} - 1} (x, y), \dots, T 2_{m_{1} - 1, 0} (x, y), \\ \dots, T 2_{m_{1} - 1, m_{2} - 1} (x, y)]^{T} . \end{matrix}

The orthogonality of LH2D-TFs set (similarly RH2D-TFs set) resulted from mutual disjointness of LH2D-TFs (and RH2D-TFs), i.e. for

i_{1}, i_{2} = 0, 1, \dots, m_{1} - 1, j_{1}, j_{2} = 0, 1, \dots, m_{2} - 1,

(14)

\int_{0}^{1} \int_{0}^{1} T 1_{i_{1}, j_{1}} (x, y) . T 2_{i_{2}, j_{2}} (x, y) = \{\begin{matrix} \frac{h_{1} h_{2}}{3}, & i_{1} = i_{2} and j_{1} = j_{2}, \\ 0, & Otherwise . \end{matrix}

The following properties of the product of two 2D-TFs vectors will be used:

(15)

\begin{matrix} T 2 (x, y) T 2^{T} (x, y) \\ ≃ (\begin{matrix} T 2_{0, 0} (x, y) & 0 & \dots & 0 \\ ⋱ & ⋮ & ⋮ & ⋮ \\ 0 & T 2_{0, m_{2} - 1} (x, y) & \dots & 0 \\ ⋮ & ⋱ & ⋮ & ⋮ \\ 0 & 0 & T 2_{m_{1} - 1, 0} (x, y) & 0 \\ ⋮ & ⋮ & ⋱ & ⋮ \\ 0 & 0 & 0 & T 2_{m_{1} - 1, m_{2} - 1} (x, y) \end{matrix}), \end{matrix}

(16)

\begin{matrix} T 1 (x, y) T 1^{T} (x, y) \\ ≃ (\begin{matrix} T 1_{0, 0} (x, y) & 0 & \dots & 0 \\ ⋱ & ⋮ & ⋮ & ⋮ \\ 0 & T 1_{0, m_{2} - 1} (x, y) & \dots & 0 \\ ⋮ & ⋱ & ⋮ & ⋮ \\ 0 & 0 & T 1_{m_{1} - 1, 0} (x, y) & 0 \\ ⋮ & ⋮ & ⋱ & ⋮ \\ 0 & 0 & 0 & T 1_{m_{1} - 1, m_{2} - 1} (x, y) \end{matrix}), \end{matrix}

and

(17)

T 1 (x, y) T 2^{T} (x, y) ≃ 0,

(18)

T 2 (x, y) T 1^{T} (x, y) ≃ 0,

where 0 is the zero

m_{1} m_{2} \times m_{1} m_{2}

matrix. To prove the above properties notice that, functions

T 1_{i, j} (x, y)

and

T 2_{i, j} (x, y)

also can be presented by

(19)

\begin{matrix} T 1_{i, j} (x, y) = u_{{ih}_{1}, {jh}_{2}} (x, y) - \frac{y - {jh}_{2}}{h_{2}} u_{{ih}_{1}, {jh}_{2}} (x, y) \\ + \frac{y - (j + 1) h_{2}}{h_{2}} u_{{ih}_{1}, (j + 1) h_{2}} (x, y), \end{matrix}

(20)

\begin{matrix} T 2_{i, j} (x, y) = \frac{y - {jh}_{2}}{h_{2}} u_{{ih}_{1}, {jh}_{2}} (x, y) - \frac{y - (j + 1) h_{2}}{h_{2}} u_{{ih}_{1}, (j + 1) h_{2}} (x, y) \\ - u_{{ih}_{1}, (j + 1) h_{2}} (x, y), \end{matrix}

for

i = 0, 1, \dots, m_{1} - 1, j = 0, 1, \dots, m_{2} - 1

, where

u_{a, b} (x, y)

is the unit step function defined as

(21)

u_{a, b} (x, y) = \{\begin{matrix} 1, & y ⩾ b, \\ 0, & y < b . \end{matrix}

Now, we show that (15) holds. For it, consider the product

T 2 (x, y) T 2^{T} (x, y)

. It is sufficient to show that for each

i = 0, 1, \dots, m_{1} - 1, j = 0, 1, \dots, m_{2} - 1

T 2_{i, j} (x, y) T 2_{i, j} (x, y) ≃ T 2_{i, j} (x, y)

. By disjointness of

T 2_{i, j} (x, y)

and

T 2_{k, t} (x, y)

for

i \neq k

and

j \neq t

the desired will be obtained:

(22)

\begin{matrix} T 2_{i, j} (x, y) T 2_{i, j} (x, y) \\ = {[\frac{y - {jh}_{2}}{h_{2}} u_{{ih}_{1}, {jh}_{2}} (x, y) - \frac{y - (j + 1) h_{2}}{h_{2}} u_{{ih}_{1}, (j + 1) h_{2}} (x, y)]}^{2} \\ - u_{{ih}_{1}, (j + 1) h_{2}} (x, y) \\ = {[\frac{y - {jh}_{2}}{h_{2}} (u_{{ih}_{1}, {jh}_{2}} (x, y) - u_{{ih}_{1}, (j + 1) h_{2}} (x, y)) + u_{{ih}_{1}, (j + 1) h_{2}} (x, y)]}^{2} \\ - u_{{ih}_{1}, (j + 1) h_{2}} (x, y) = {(\frac{y - {jh}_{2}}{h_{2}})}^{2} (u_{{ih}_{1}, {jh}_{2}} (x, y) - u_{{ih}_{1}, (j + 1) h_{2}} (x, y)) \\ ≃ T 2_{i, j} (x, y) . \end{matrix}

Eq. (16) can be obtained similarly. Notice that, we can approximate the result by

T 1_{i, j} (x, y)

and

T 2_{i, j} (x, y)

{(\frac{y - {jh}_{2}}{h_{2}})}^{2} (u_{{ih}_{1}, {jh}_{2}} (x, y) - u_{{ih}_{1}, (j + 1) h_{2}} (x, y))

is possibly nonzero only in

[{jh}_{2}, (j + 1) h_{2})

. Thus, the last relation in (22) is followed.

We now show that Eq. (17) holds. For it

(23)

\begin{matrix} T 1_{i, j} (x, y) T 2_{i, j} (x, y) = T 2_{i, j} (x, y) - [\frac{y - {jh}_{2}}{h_{2}} u_{{ih}_{1}, {jh}_{2}} (x, y) \\ {- \frac{y - (j + 1) h_{2}}{h_{2}} u_{{ih}_{1}, (j + 1) h_{2}} (x, y)]}^{2} \\ + u_{{ih}_{1}, (j + 1) h_{2}} (x, y) . \end{matrix}

Similar argument discussed for (22) results that

(24)

\begin{matrix} T 1_{i, j} (x, y) T 2_{i, j} (x, y) ≃ T 2_{i, j} (x, y) - (T 2_{i, j} (x, y) + u_{{ih}_{1}, (j + 1) h_{2}} (x, y)) \\ + u_{{ih}_{1}, (j + 1) h_{2}} (x, y) ≃ 0, \end{matrix}

thus,

(25)

T 1_{i, j} (x, y) T 2_{i, j} (x, y) = T 2_{i, j} (x, y) T 1_{i, j} (x, y) ≃ 0,

where

0 \in R .

3.3

3.3 2D-TFs expansion

We can approximate the function $f (x, y) \in L^{2} ([0, 1) \times [0, 1))$ by 2D-TFs as follows: $\begin{matrix} f (x, y) ≃ [c_{0, 0} T 1_{0, 0} + c_{0, 1} T 2_{0, 0}] + \dots + [c_{0, m_{2} - 1} T 1_{0, m_{2} - 1} \\ + c_{0, m_{2}} T 2_{0, m_{2} - 1}] + \dots + [c_{m_{1} - 1, 0} T 1_{m_{1} - 1, 0} + c_{m_{1} - 1, 1} T 2_{m_{1} - 1, 0}] \\ + \dots + [c_{m_{1} - 1, m_{2} - 1} T 1_{m_{1} - 1, m_{2} - 1} + c_{m_{1} - 1, m_{2}} T 2_{m_{1} - 1, m_{2} - 1}] \\ = [c_{0, 0}, \dots, c_{0, m_{2} - 1}, \dots, c_{m_{1} - 1, 0}, \dots, c_{m_{1} - 1, m_{2} - 1}] T 1 (x, y) \\ + [c_{0, 1}, \dots, c_{0, m_{2}}, \dots, c_{m_{1} - 1, 1}, \dots, c_{m_{1} - 1, m_{2}}] T 2 (x, y), \end{matrix}$ thus

(26)

f (x, y) ≃ \sum_{i = 0}^{m_{1} - 1} \sum_{j = 0}^{m_{2} - 1} c_{i, j} T 1_{i, j} (x, y) + \sum_{i = 0}^{m_{1} - 1} \sum_{j = 0}^{m_{2} - 1} c_{i, j + 1} T 2_{i, j} (x, y),

where

(27)

\{\begin{matrix} c_{i, j} = f ({ih}_{1}, {jh}_{2}), \\ c_{i, j + 1} = f ({ih}_{1}, (j + 1) h_{2}) . \end{matrix}

Preference

Preference (Maleknejad et al., 2010)

It is apparent from (6) and (27) that unlike 2D-BPFs the representation by 2D-TFs does not need any integration to evaluate the coefficients, there by reducing a lot of computational efforts.

3.3.1

3.3.1 Expanding four variables function by 2D-TFs

We can expand $K (x, y, t, s) \in L^{2} ([0, 1) \times [0, 1) \times [0, 1) \times [0, 1))$ by 2D-TFs vectors, with $m_{1} m_{2}$ and $m_{3} m_{4}$ components, respectively. For convenience, consider $m = m_{1} = m_{2} = m_{3} = m_{4} .$ For obtaining desired results, we first fix the independent variables t, s. Then, expand $K (x, y, t, s)$ by 2D-TFs with respect to independent variables x, y as follows: $\begin{matrix} K (x, y, t, s) ≃ T 1^{T} (x, y) (\begin{matrix} K (0, 0, t, s) \\ ⋮ \\ K (0, (m - 1) h, t, s) \\ ⋮ \\ K ((m - 1) h, 0, t, s) \\ ⋮ \\ K ((m - 1) h, (m - 1) h, t, s) \end{matrix}) \\ + T 2^{T} (x, y) (\begin{matrix} K (0, h, t, s) \\ ⋮ \\ K (0, mh, t, s) \\ ⋮ \\ K ((m - 1) h, h, t, s) \\ ⋮ \\ K ((m - 1) h, mh, t, s) \end{matrix}) . \end{matrix}$ Now each of $K (ih, jh, t, s) s$ for $i, j = 0, 1, \dots, m - 1$ can be expanded by 2D-TFs with respect to independent variables t, s. Hence, the expansion of $K (x, y, t, s)$ can be written as $\begin{matrix} K (x, y, t, s) ≃ T 1^{T} (x, y) (\begin{matrix} K 11_{1}^{T} T 1 (t, s) + K 12_{1}^{T} T 2 (t, s) \\ ⋮ \\ K 11_{m}^{T} T 1 (t, s) + K 12_{m}^{T} T 2 (t, s) \\ ⋮ \\ K 11_{m (m - 1)}^{T} T 1 (t, s) + K 12_{m (m - 1)}^{T} T 2 (t, s) \\ ⋮ \\ K 11_{m^{2}}^{T} T 1 (t, s) + K 12_{m^{2}}^{T} T 2 (t, s) \end{matrix}) \\ + T 2^{T} (x, y) (\begin{matrix} K 21_{1}^{T} T 1 (t, s) + K 22_{1}^{T} T 2 (t, s) \\ ⋮ \\ K 21_{m}^{T} T 1 (t, s) + K 22_{m}^{T} T 2 (t, s) \\ ⋮ \\ K 21_{m (m - 1)}^{T} T 1 (t, s) + K 22_{m (m - 1)}^{T} T 2 (t, s) \\ ⋮ \\ K 21_{m^{2}}^{T} T 1 (t, s) + K 22_{m^{2}}^{T} T 2 (t, s) \end{matrix}) \\ = T 1^{T} (x, y) K 11 T 1 (t, s) + T 1^{T} (x, y) K 12 T 2 (t, s) \\ + T 2^{T} (x, y) K 21 T 1 (t, s) + T 2^{T} (x, y) K 22 T 2 (t, s), \end{matrix}$ which in equation above

(28)

K 11 = (\begin{matrix} {(\begin{matrix} K (0, 0, 0, 0) \\ ⋮ \\ K (0, 0, 0, (m - 1) h) \\ ⋮ \\ K (0, 0, (m - 1) h, 0) \\ ⋮ \\ K (0, 0, (m - 1) h, (m - 1) h) \end{matrix})}^{T} \\ ⋮ \\ {(\begin{matrix} K (0, (m - 1) h, 0, 0) \\ ⋮ \\ K (0, (m - 1) h, 0, (m - 1) h) \\ ⋮ \\ K (0, (m - 1) h, (m - 1) h, 0) \\ ⋮ \\ K (0, (m - 1) h, (m - 1) h, (m - 1) h) \end{matrix})}^{T} \\ ⋮ \\ {(\begin{matrix} K ((m - 1) h, 0, 0, 0) \\ ⋮ \\ K ((m - 1) h, 0, 0, (m - 1) h) \\ ⋮ \\ K ((m - 1) h, 0, (m - 1) h, 0) \\ ⋮ \\ K ((m - 1) h, 0, (m - 1) h, (m - 1) h) \end{matrix})}^{T} \\ ⋮ \\ {(\begin{matrix} K ((m - 1) h, (m - 1) h, 0, 0) \\ ⋮ \\ K ((m - 1) h, (m - 1) h, 0, (m - 1) h) \\ ⋮ \\ K ((m - 1) h, (m - 1) h, (m - 1) h, 0) \\ ⋮ \\ K ((m - 1) h, (m - 1) h, (m - 1) h, (m - 1) h) \end{matrix})}^{T} \end{matrix}), K 12 = (\begin{matrix} {(\begin{matrix} K (0, 0, 0, h) \\ ⋮ \\ K (0, 0, 0, mh) \\ ⋮ \\ K (0, 0, (m - 1) h, h) \\ ⋮ \\ K (0, 0, (m - 1) h, mh) \end{matrix})}^{T} \\ ⋮ \\ {(\begin{matrix} K (0, (m - 1) h, 0, h) \\ ⋮ \\ K (0, (m - 1) h, 0, mh) \\ ⋮ \\ K (0, (m - 1) h, (m - 1) h, h) \\ ⋮ \\ K (0, (m - 1) h, (m - 1) h, mh) \end{matrix})}^{T} \\ ⋮ \\ {(\begin{matrix} K ((m - 1) h, 0, 0, h) \\ ⋮ \\ K ((m - 1) h, 0, 0, mh) \\ ⋮ \\ K ((m - 1) h, 0, (m - 1) h, h) \\ ⋮ \\ K ((m - 1) h, 0, (m - 1) h, mh) \end{matrix})}^{T} \\ ⋮ \\ {(\begin{matrix} K ((m - 1) h, (m - 1) h, 0, h) \\ ⋮ \\ K ((m - 1) h, (m - 1) h, 0, mh) \\ ⋮ \\ K ((m - 1) h, (m - 1) h, (m - 1) h, h) \\ ⋮ \\ K ((m - 1) h, (m - 1) h, (m - 1) h, mh) \end{matrix})}^{T} \end{matrix}),

(29)

K 21 = (\begin{matrix} {(\begin{matrix} K (0, h, 0, 0) \\ ⋮ \\ K (0, h, 0, (m - 1) h) \\ ⋮ \\ K (0, h, (m - 1) h, 0) \\ ⋮ \\ K (0, h, (m - 1) h, (m - 1) h) \end{matrix})}^{T} \\ ⋮ \\ {(\begin{matrix} K (0, mh, 0, 0) \\ ⋮ \\ K (0, mh, 0, (m - 1) h) \\ ⋮ \\ K (0, mh, (m - 1) h, 0) \\ ⋮ \\ K (0, mh, (m - 1) h, (m - 1) h) \end{matrix})}^{T} \\ ⋮ \\ {(\begin{matrix} K ((m - 1) h, h, 0, 0) \\ ⋮ \\ K ((m - 1) h, h, 0, (m - 1) h) \\ ⋮ \\ K ((m - 1) h, h, (m - 1) h, 0) \\ ⋮ \\ k ((m - 1) h, h, (m - 1) h, (m - 1) h) \end{matrix})}^{T} \\ ⋮ \\ {(\begin{matrix} K ((m - 1) h, mh, 0, 0) \\ ⋮ \\ K ((m - 1) h, mh, 0, (m - 1) h) \\ ⋮ \\ K ((m - 1) h, mh, (m - 1) h, 0) \\ ⋮ \\ K ((m - 1) h, mh, (m - 1) h, (m - 1) h) \end{matrix})}^{T} \end{matrix}), K 22 = (\begin{matrix} {(\begin{matrix} K (0, h, 0, h) \\ ⋮ \\ K (0, h, 0, mh) \\ ⋮ \\ K (0, h, (m - 1) h, h) \\ ⋮ \\ K (0, h, (m - 1) h, mh) \end{matrix})}^{T} \\ ⋮ \\ {(\begin{matrix} K (0, mh, 0, h) \\ ⋮ \\ K (0, mh, 0, mh) \\ ⋮ \\ K (0, mh, (m - 1) h, h) \\ ⋮ \\ K (0, mh, (m - 1) h, mh) \end{matrix})}^{T} \\ ⋮ \\ {(\begin{matrix} K ((m - 1) h, h, 0, h) \\ ⋮ \\ K ((m - 1) h, h, 0, mh) \\ ⋮ \\ K ((m - 1) h, h, (m - 1) h, h) \\ ⋮ \\ K ((m - 1) h, h, (m - 1) h, mh) \end{matrix})}^{T} \\ ⋮ \\ {(\begin{matrix} K ((m - 1) h, mh, 0, h) \\ ⋮ \\ K ((m - 1) h, mh, 0, mh) \\ ⋮ \\ K ((m - 1) h, mh, (m - 1) h, h) \\ ⋮ \\ K ((m - 1) h, mh, (m - 1) h, mh) \end{matrix})}^{T} \end{matrix}),

3.4

3.4 Other properties

Other properties that we will need are

(30)

\begin{matrix} \int_{0}^{1} \int_{0}^{1} T 1 (x, y) . T 1^{T} (x, y) dy dx \\ = \int_{0}^{1} \int_{0}^{1} T 2 (x, y) . T 2^{T} (x, y) dy dx = \frac{h_{1} h_{2}}{3} I, \end{matrix}

(31)

\begin{matrix} \int_{0}^{1} \int_{0}^{1} T 1 (x, y) . T 2^{T} (x, y) dy dx \\ = \int_{0}^{1} \int_{0}^{1} T 2 (x, y) . T 1^{T} (x, y) dy dx = \frac{h_{1} h_{2}}{6} I, \end{matrix}

where I is

m_{1} m_{2} \times m_{1} m_{2}

identity matrix. To show (30), consider (22) again

(32)

\int_{0}^{1} \int_{0}^{1} T 2 (x, y) . T 2^{T} (x, y) dy dx = \int_{{ih}_{1}}^{(i + 1) h_{1}} \int_{{jh}_{2}}^{(j + 1) h_{2}} {(\frac{y - {jh}_{2}}{h_{2}})}^{2} (u_{{ih}_{1}, {jh}_{2}} (x, y) - u_{{ih}_{1}, (j + 1) h_{2}} (x, y)) dy dx = \int_{{ih}_{1}}^{(i + 1) h_{1}} \int_{{jh}_{2}}^{(j + 1) h_{2}} {(\frac{y - {jh}_{2}}{h_{2}})}^{2} dy dx = \int_{{ih}_{1}}^{(i + 1) h_{1}} \frac{h_{2}}{3} dx = \frac{h_{1} h_{2}}{3} .

Similarly,

\int_{0}^{1} \int_{0}^{1} T 1 (x, y) . T 1^{T} (x, y) dy dx = \frac{h_{1} h_{2}}{3} .

Now

(33)

\int_{0}^{1} \int_{0}^{1} T 1 (x, y) . T 2^{T} (x, y) dy dx = \int_{{ih}_{1}}^{(i + 1) h_{1}} \int_{{jh}_{2}}^{(j + 1) h_{2}} (1 - \frac{y - {jh}_{2}}{h_{2}}) (\frac{y - {jh}_{2}}{h_{2}}) dy dx = \int_{{ih}_{1}}^{(i + 1) h_{1}} \int_{{jh}_{2}}^{(j + 1) h_{2}} \frac{y - {jh}_{2}}{h_{2}} dy dx - \int_{{ih}_{1}}^{(i + 1) h_{1}} \int_{{jh}_{2}}^{(j + 1) h_{2}} {(\frac{y - {jh}_{2}}{h_{2}})}^{2} dy dx = \frac{h_{1} h_{2}}{2} - \frac{h_{1} h_{2}}{3} = \frac{h_{1} h_{2}}{6} .

Similarly,

\int_{0}^{1} \int_{0}^{1} T 2 (x, y) . T 1^{T} (x, y) dy dx = \frac{h_{1} h_{2}}{6} .

4 Two-dimensional Fredholm integral equation of the second kind

In this section, we present a 2D-TFs method for solving Eq. (1). Changing the variables $τ = (b - a) x + a, μ = (d - c) y + c, λ = (b - a) t + a$ and $η = (d - c) s + c,$ Eq. (1) can be written as

(34)

u (x, y) = f (x, y) + (b - a) (d - c) \int_{0}^{1} \int_{0}^{1} K (x, y, t, s) u (t, s) dt ds; (x, y) \in D,

where

\begin{matrix} f (x, y) = f_{1} ((b - a) x + a, (d - c) y + c), \\ K (x, y, t, s) = K_{1} ((b - a) x + a, (d - c) y \\ + c, (b - a) t + a, (d - c) s + c), \\ u (x, y) = u_{1} ((b - a) x + a, (d - c) y + c), \end{matrix}

and

D = L^{2} ([0, 1) \times [0, 1))

Let us expand $f (x, y)$ and $u (x, y)$ by 2D-TFs (LH2D-TF and RH2D-TF) as follows:

(35)

f (x, y) ≃ F_{1}^{T} T 1 (x, y) + F_{2}^{T} T 2 (x, y),

(36)

u (x, y) ≃ U_{1}^{T} T 1 (x, y) + U_{2}^{T} T 2 (x, y) .

As described in Section 3.3.1, we can expand

K (x, y, t, s)

in the interval

L^{2} ([0, 1) \times [0, 1) \times [0, 1) \times [0, 1))

by 2D-TF. Suppose that this approximation be as follows:

(37)

K (x, y, t, s) ≃ T 1^{T} (x, y) K 11 T 1 (t, s) + T 1^{T} (x, y) K 12 T 2 (t, s) + T 2^{T} (x, y) K 21 T 1 (t, s) + T 2^{T} (x, y) K 22 T 2 (t, s),

where

K 11, K 12, K 21

and K22 are obtained from Eqs. (28) and (29). Then, we have

U_{1}^{T} T 1 (x, y) + U_{2}^{T} T 2 (x, y) = F_{1}^{T} T 1 (x, y) + F_{2}^{T} T 2 (x, y) + ρ \int_{0}^{m_{1} h_{1}} \int_{0}^{m_{2} h_{2}} (U_{1}^{T} T 1 (t, s) + U_{2}^{T} T 2 (t, s)) [T 1^{T} (x, y) K 11 T 1 (t, s) + T 1^{T} (x, y) K 12 T 2 (t, s) + T 2^{T} (x, y) K 21 T 1 (t, s) + T 2^{T} (x, y) K 22 T 2 (t, s)] ds dt,

where

ρ = (b - a) (d - c)

. Thus, we have

\begin{matrix} U_{1}^{T} T 1 (x, y) + U_{2}^{T} T 2 (x, y) \\ = F_{1}^{T} T 1 (x, y) + F_{2}^{T} T 2 (x, y) \\ + ρ [U_{1}^{T} \int_{0}^{m_{1} h_{1}} \int_{0}^{m_{2} h_{2}} T 1 (t, s) T 1^{T} (t, s) K 11^{T} T 1 (x, y) dt ds \\ + U_{1}^{T} \int_{0}^{m_{1} h_{1}} \int_{0}^{m_{2} h_{2}} T 1 (t, s) T 2^{T} (t, s) K 12^{T} T 1 (x, y) dt ds \\ + U_{1}^{T} \int_{0}^{m_{1} h_{1}} \int_{0}^{m_{2} h_{2}} T 1 (t, s) T 1^{T} (t, s) K 21^{T} T 2 (x, y) dt ds \\ + U_{1}^{T} \int_{0}^{m_{1} h_{1}} \int_{0}^{m_{2} h_{2}} T 1 (t, s) T 2^{T} (t, s) K 22^{T} T 2 (x, y) dt ds \\ + U_{2}^{T} \int_{0}^{m_{1} h_{1}} \int_{0}^{m_{2} h_{2}} T 2 (t, s) T 1^{T} (t, s) K 11^{T} T 1 (x, y) dt ds \\ + U_{2}^{T} \int_{0}^{m_{1} h_{1}} \int_{0}^{m_{2} h_{2}} T 2 (t, s) T 2^{T} (t, s) K 12^{T} T 1 (x, y) dt ds \\ + U_{2}^{T} \int_{0}^{m_{1} h_{1}} \int_{0}^{m_{2} h_{2}} T 2 (t, s) T 1^{T} (t, s) K 21^{T} T 2 (x, y) dt ds \\ + U_{2}^{T} \int_{0}^{m_{1} h_{1}} \int_{0}^{m_{2} h_{2}} T 2 (t, s) T 2^{T} (t, s) K 22^{T} T 2 (x, y) dt ds] . \end{matrix}

By using definite integral formula for 2D-TFs in (30) and (31), we have

(38)

U_{1}^{T} T 1 (x, y) + U_{2}^{T} T 2 (x, y) = F_{1}^{T} T 1 (x, y) + F_{2}^{T} T 2 (x, y) + ρ [U_{1}^{T} (\frac{h_{1} h_{2}}{3} K 11^{T} T 1 (x, y) + \frac{h_{1} h_{2}}{6} K 12^{T} T 1 (x, y) + \frac{h_{1} h_{2}}{3} K 21^{T} T 2 (x, y) + \frac{h_{1} h_{2}}{6} K 22^{T} T 2 (x, y)) + U_{2}^{T} (\frac{h_{1} h_{2}}{6} K 11^{T} T 1 (x, y) + \frac{h_{1} h_{2}}{3} K 12^{T} T 1 (x, y) + \frac{h_{1} h_{2}}{6} K 21^{T} T 2 (x, y) + \frac{h_{1} h_{2}}{3} K 22^{T} T 2 (x, y))] .

The coefficients of

T 1 (x, y)

and

T 2 (x, y)

on both sides of (38) must be equal; hence, we have the following equations for the corresponding coefficients of 2D-TFs:

\{\begin{matrix} U_{1}^{T} (I - ρ (\frac{h_{1} h_{2}}{3} K 11^{T} + \frac{h_{1} h_{2}}{6} K 12^{T})) \\ - ρ U_{2}^{T} (\frac{h_{1} h_{2}}{6} K 11^{T} + \frac{h_{1} h_{2}}{3} K 12^{T}) = F_{1}^{T} \\ - ρ U_{1}^{T} (\frac{h_{1} h_{2}}{3} K 21^{T} + \frac{h_{1} h_{2}}{6} K 22^{T}) \\ + U_{2}^{T} (I - ρ (\frac{h_{1} h_{2}}{6} K 21^{T} + \frac{h_{1} h_{2}}{3} K 22^{T})) = F_{2}^{T} \end{matrix} .

Set

(39)

A_{1} = I - ρ (\frac{h_{1} h_{2}}{3} K 11 + \frac{h_{1} h_{2}}{6} K 12),

(40)

A_{2} = - ρ (\frac{h_{1} h_{2}}{6} K 11 + \frac{h_{1} h_{2}}{3} K 12),

(41)

B_{1} = - ρ (\frac{h_{1} h_{2}}{3} K 21 + \frac{h_{1} h_{2}}{6} K 22),

(42)

B_{2} = I - ρ (\frac{h_{1} h_{2}}{6} K 21 + \frac{h_{1} h_{2}}{3} K 22) .

Then, we have the following linear system:

(43)

(\begin{matrix} A_{1} & A_{2} \\ B_{1} & B_{2} \end{matrix}) (\begin{matrix} U_{1} \\ U_{2} \end{matrix}) = (\begin{matrix} F_{1} \\ F_{2} \end{matrix}) .

After solving the above linear system, we can find

U_{1}

and

U_{2}

and then

(44)

u (x, y) ≃ U_{1}^{T} T 1 (x, y) + U_{2}^{T} T 2 (x, y),

is the estimation of the solution of the two-dimensional Fredholm integral equation of the second kind.

5 Convergence analysis

Assume $(C [J], ‖ . ‖)$ the Banach space of all continuous functions on $J = [0, 1) \times [0, 1)$ with norm $‖ f (x, y) ‖ = \max_{\forall (x, y) \in J} | f (x, y) |$ .

Let $\forall x, y, t, s \in [0, 1), | k (x, y, s, t) | ⩽ M$ . We denote the error 2D-TFS by $e_{2D-TFS} = ‖ u_{m} (x, y) - u (x, y) ‖,$ where $u_{m} (x, y)$ and $u (x, y)$ show the approximate and exact solutions of the two-dimensional linear Fredholm integral equation, respectively. If we note to Eq. (27), we will see the coefficients $c_{i, j}$ 's and $d_{i, j + 1}$ 's are not optimal. By using the optimal coefficients, the representational errors $e_{2 D - TFS}$ can be reduced.

Theorem 1

The solution of the two-dimensional linear Fredholm integral equation by using 2D-TFs approximation converges if $0 < α < 1 .$

Proof

Let $\begin{matrix} ‖ u_{m} (x, y) - u (x, y) ‖ \\ = \max_{\forall (x, y) \in J} | u_{m} (x, y) - u (x, y) | \\ = \max |f (x, y) + ρ \int_{0}^{1} \int_{0}^{1} k (x, y, t, s) u_{m} (t, s) dt ds - f (x, y) \\ - ρ \int_{0}^{1} \int_{0}^{1} k (x, y, t, s) u (t, s) dt ds| \\ ⩽ \max | ρ | \int_{0}^{1} \int_{0}^{1} | k (x, y, t, s) | | u_{m} (t, s) - u (t, s) | dt ds \\ ⩽ | ρ | M \int_{0}^{1} \int_{0}^{1} \max | u_{m} (t, s) - u (t, s) | dt ds \\ = | ρ | M ‖ u_{m} (x, y) - u (x, y) ‖, \end{matrix}$ $\Rightarrow ‖ u_{m} (x, y) - u (x, y) ‖ ⩽ α ‖ u_{m} (x, y) - u (x, y) ‖,$ where $α = | ρ | M .$

We get $(1 - α) ‖ u_{m} (x, y) - u (x, y) ‖ ⩽ 0$ and choose $0 < α < 1$ , by increasing m, it implies $‖ u_{m} (x, y) - u (x, y) ‖ \to 0$ as $m \to \infty$ . □

6 Numerical illustration

In this section, we present one example and their numerical results to show the high accuracy of the solution obtained by 2D-TFs.

Example 5.1

Consider the two-dimensional linear Fredholm integral equation

(45)

u (x, y) = g (x, y) + \int_{0}^{1} \int_{0}^{1} \frac{x}{(8 + y) (1 + t + s)} u (t, s) dt ds,

where

(x, y) \in [0, 1) \times [0, 1)

and

g (x, y) = \frac{1}{(1 + x + y)^{2}} - \frac{x}{6 (8 + y)} .

It's exact solution is

u (x, y) = \frac{1}{(1 + x + y)^{2}}

. The solution for

u (x, y)

is obtained by 2D-TFs method described in Section 4, for

m = 32

is collected as shown in Table 1.

Table 1 Numerical results of example 1 with 2D-TFs.

Nodes (x, y)	2D-TFs method with m = 32	Error of 2D-TFs method with m = 32	Exact solution
(0, 0)	1.0000e−000	0	1.0000e−000
(0.1, 0.1)	7.020e−001	−7.5800e−003	6.9444e−001
(0.2, 0.2)	5.1974e−001	−9.5400e−003	5.1020e−001
(0.3, 0.3)	4.0022e−001	−9.6000e−003	3.9062e−001
(0.4, 0.4)	3.1767e−001	−9.0300e−003	3.0864e−001
(0.5, 0.5)	2.5030e−001	−3.0000e−004	2.5000e−001
(0.6, 0.6)	2.0704e−001	−4.3000e−004	2.0661e−001
(0.7, 0.7)	1.7585e−001	−2.2400e−003	1.7361e−001
(0.8, 0.8)	1.5055e−001	−2.6200e−003	1.4793e−001
(0.9, 0.9)	1.3036e−001	−2.8100e−003	1.2755e−001

7 Conclusion

Two-dimensional Fredholm integral equations are usually difficult to solve analytically. In many cases, it is required to obtain the approximate solutions, for this purpose the presented method can be proposed. We have investigated the application of orthogonal functions by 2D-TFs for solving the linear two-dimensional Fredholm integral equations. This technique is very simple and involves less computation. Also we can expand this method to higher dimensional problems and other classes of integral equations such as nonlinear two-dimensional Fredholm integral equations, linear and nonlinear two-dimensional Volterra integral equations.

References

Alipanah, A., Esmaeili, S., 2009. Numerical solution of the two-dimensional Fredholm integral equations using Gaussian radial basis function. J. Comput. Appl. Math., in press.
Alpert B., Beylkin G., Coifman R., Rokhlin V., . Wavelets for the fast solution of second-kind integral equations. SIAM J. Sci. Comput.. 1993;14:159-184.
[Google Scholar]
Anselon P.M., . Collectively Compact Operator Approximation Theory. Englewood Cliffs, NJ: Prentice-Hall; 1971.
Atkinson K.E., . A Survey of Numerical Methods for the Solution of Fredholm Integral Equations of the Second Kind. Philadelphia, PA: SIAM; 1976.
Atkinson K.E., . The Numerical Solution of Integral Equations of the Second Kind. Cambridge: Cambridge University Press; 1997.
Babolian E., Marzban H.R., Salmani M., . Using triangular orthogonal functions for solving Fredholm integral equations of the second kind. Appl. Math. Comput.. 2008;201:452-456.
[Google Scholar]
Baker C.T., . The Numerical Treatment of Integral Equations. Oxford: Clarendon Press; 1977.
Deb A., Dasgupta A., Sarkar G., . A new set of orthogonal functions and its application to the analysis of dynamic systems. J. Franklin Inst.. 2006;343:1-26.
[Google Scholar]
Delves L.M., Mohamed J.L., . Computational Method for Integral Equations. New York: Cambridge University Press; 1985.
Farengo R., Lee Y.C., Guzdar P.N., . An electromagnetic integral equation: application to microtearing modes. Phys. Fluids. 1983;26:3515-3523.
[Google Scholar]
Maleknejad K., Sohrabi S., Baranji B., . Application of 2D-BPFs to nonlinear integral equations. Commun. Nonlinear Sci. Numer. Simulat.. 2010;15:527-532.
[Google Scholar]