Translate this page into:
A Riccati-type solution of 3D Euler equations for incompressible flow
⁎Corresponding author. sergej-ershkov@yandex.ru (Sergey V. Ershkov),
-
Received: ,
Accepted: ,
This article was originally published by Elsevier and was migrated to Scientific Scholar after the change of Publisher.
Peer review under responsibility of King Saud University.
Abstract
In fluid mechanics, a lot of authors have been reporting analytical solutions of Euler and Navier-Stokes equations. But there is an essential deficiency of non-stationary solutions indeed.
In our presentation, we explore the case of non-stationary flows of the Euler equations for incompressible fluids, which should conserve the Bernoulli-function to be invariant for the aforementioned system.
We use previously suggested ansatz for solving of the system of Navier-Stokes equations (which is proved to have the analytical way to present its solution in case of conserving the Bernoulli-function to be invariant for such the type of the flows). Conditions for the existence of exact solution of the aforementioned type for the Euler equations are obtained. The restrictions at choosing of the form of the 3D nonstationary solution for the given constant Bernoulli-function B are considered. We should especially note that pressure field should be calculated from the given constant Bernoulli-function B, if all the components of velocity field are obtained.
Keywords
Euler equations
Bernoulli-function
Non-stationary solutions
1 Introduction the Euler system of equations
The Euler system of equations for incompressible flow of inviscid fluid is known to be one of the old famous problems in classical fluid mechanics, besides we should especially note that a lot of great scientists have been trying to solve such a problem during last 265 years.
In accordance with (Landau and Lifshitz, 1987; Ladyzhenskaya, 1969; Lighthill, 1986), the Euler system of equations for incompressible flow of inviscid fluid should be presented in the Cartesian coordinates as below (under the proper initial or boundary conditions):
2 The originating system of PDE for Euler Eqs
Using the identity (u·∇)u = (1/2)∇(u2) − u×(∇ × u), we could present Eqs. (1)–(2) in case of incompressible flow u = {u1, u2, u3} as below (Saffman, 1995; Milne-Thomson, 1950):
-
Steady inviscid flow (the solution does not depend on time);
-
Irrotational flow (the fluid particles don't spin, i.e., the curl of velocity is zero).
3 Presentation of the time-dependent part of solution
The second of the equations of system (3) with the additional demand (5) is proved to have the analytical way to present its solution (Ershkov, 2015, 2016, 2017) in a form below (in regard to the time-parameter t):
It is clear that for the correct solving of system (3) with additional demand (5) we should obtain from Eqs. (4), (6) the three additional equations below (which should determine the spatial part of the curl field w):
Thus, let us search for the exact solution in a form (11); in this case, expressions for the components of the curl field (8) should be presented as below:
which, taking into account expressions (10)–(11), should be transformed to the form below (γ2 > σ2):
Obviously, system of Eqs. (14) is the system of two non-linear ordinary differential equations of the 1-st order for two functions A(t), σ(t) (which could be solved by numerical methods only).
But nevertheless, the solution of system (14) exists, that’s why solution of the system of Eqs. (3) should exist also (for the presented, previously chosen form of the solution (5) + (6)). Approximate solutions of (14) are presented in the next section.
4 Final presentation of the solution
Let us present the non-stationary exact solution {p, u} of the Euler Eqs. (3) in its final form, which should conserve the Bernoulli-function (B = const) (5) to be invariant for the aforementioned system:
Let us especially note that function γ(x,y,z) along with the functions {u1, u2, u3} are proved to satisfy to the continuity Eq. (1) for such an expressions.
System of Eqs. (14) could be transformed as below (let us note again that we consider variables {x,y,z} as variable parameters):
or, it can be transformed properly
Let us schematically imagine at Figs. 1–3 the appropriate components of velocity field {u1, u2, u3}, according to the formulae (15), which correspond to the approximate solutions (20) for functions A(t), σ(t) via derivation (18)–(19):
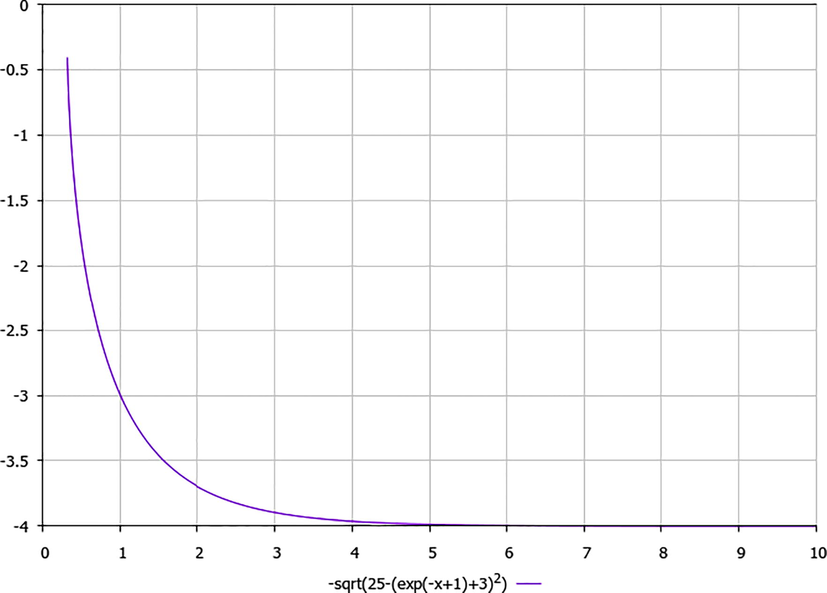
A schematic plot of the component u1 (t) of velocity field (15), depending on time t (for simplicity, B = 1).

A schematic plot of the component u2 (t) of velocity field (15), depending on time t (for simplicity, B = 1).
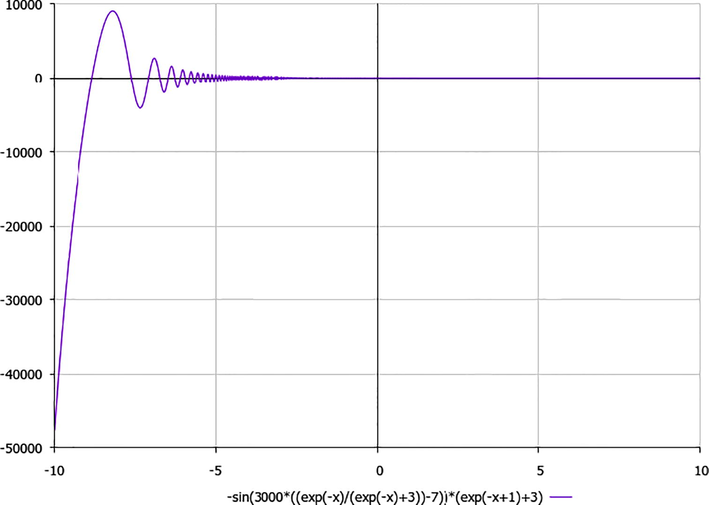
A schematic plot of the component u3 (t) of velocity field (15), depending on time t (for simplicity, B = 1).
5 Discussion and conclusion
Meanwhile, the intriguing fact is that Riccati-type seems to be the specific feature for a lot of the solutions in fluid mechanics and magneto-hydrodynamics (Christianto and Smarandache, 2008; Gibbon et al., 2006). We should mention very interesting results of article (Gibbon, 2002), where author discusses the presentation of components of the time-dependent solution for 3D Euler equation, which are supposed to be associated with complex Riccati equation via quaternionic formulation.
We should also mention the paper (Wilchynski, 2009), in which author provides an example of the Riccati equation without periodic solutions which appears in the Euler vorticity dynamics.
All in all, Euler and Navier-Stokes equations have already been investigated in many researches including their numerical and analytical solutions (Drazin and Riley, 2006). However essential deficiency exists in the studies of non-stationary solutions of even the Euler equations for ideal, inviscid flow.
In this derivation, we explore the case of non-stationary flows of the Euler equations for incompressible fluids, which should conserve the Bernoulli-function for the flow.
The aforementioned approach quite differs from that which was used previously in investigation of Euler equations (Ershkov, 2015) by one of the authors. Indeed, an exuberant assumption was made in (Ershkov, 2015), such as separating of variables for the presenting of each components of the solution (including the pressure field). As for the relevance of such the purely mathematical assumption, it seems not to be physically reasonable if we will compare it with the approach for obtaining of our new solution with constant Bernoulli-function which should be invariant inside the limited domain of the flow.
To obtain new solution, we use previously suggested ansatz for solving of the system of Navier-Stokes equations (which is proved to have the analytical way to present its solution in case of conserving the Bernoulli-function for this type of the flows). Conditions for the existence of exact solution of the aforementioned type are proposed. The restrictions at choosing of the form of 3D solution for the given constant Bernoulli-function B are emphasized: for example, the pressure field should be calculated from the given constant Bernoulli-function B, if all the components of velocity field are obtained.
Besides, we should especially note that in our case of solution, we need some boundary conditions that preserve the aforementioned solution inside of the limited domain: indeed, the pressure field should strongly depend on the spatial part of the initial conditions for the components of velocity field (according to the Bernoulli invariant).
Also we should note that since the fluid is incompressible for the development above, there is a strong link between boundary conditions and the solution inside.
The uniqueness of the presented solutions is not considered. In this respect we confine ourselves to mention the paper (Galdi and Rionero, 1979), in which all the difficulties concerning the uniqueness in unbounded domain are remarked (which should be the same for analytical solutions in inviscid fluids if the kinematic viscosity tends to zero).
The last but not least, we have found an elegant way to simplify the presented solution up to the analytical presentation of the approximate solution. Also it has to be specified that the solutions that are constructed can be considered as a class of perturbation of a solutions, absorbed exponentially as t going to infinity ∞ by the null solution.
Acknowledgements
Authors are thankful to Dr. A.V. Koptev (Koptev, 2014), to Dr. V.I. Semenov (Semenov, 2014), and especially to Dr. G.V. Alekseev (Alekseev, 2016) with respect to useful discussions during preparing of the PhD-thesis by Sergey V. Ershkov as well as for their wise advices regarding the further works (including this manuscript).
We should also appreciate the efforts of 3 esteemed reviewers for their valuable advices which improved structure of the article significantly. This study was initiated in the framework of the state task programme in the sphere of scientific activity of the Ministry of Education and Science of the Russian Federation (project No. 5.5176.2017/8.9) and grant of the President of the Russian Federation for state support of the leading scientific schools of the Russian Federation (NSh-6637.2016.5).
References
- Solvability of an inhomogeneous boundary value problem for the stationary magnetohydrodynamic equations for a viscous incompressible fluid. Diff. Equ.. 2016;52(6):739-748.
- [Google Scholar]
- An exact mapping from Navier-Stokes equation to Schroedinger equation. Progress Phys.. 2008;1:38-39.
- [Google Scholar]
- The Navier-Stokes Equations: A Classification of Flows and Exact Solutions. Cambridge: Cambridge University Press; 2006.
- Non-stationary Riccati-type flows for incompressible 3D Navier-Stokes equations. Comput. Math. Appl.. 2015;71(7):1392-1404.
- [Google Scholar]
- A procedure for the construction of non-stationary Riccati-type flows for incompressible 3D Navier-Stokes equations. Rendiconti del Circolo Matematico di Palermo. 2016;65(1):73-85.
- [Google Scholar]
- A Riccati-type solution of Euler-Poisson equations of rigid body rotation over the fixed point. Acta Mech.. 2017;228(7):2719-2723.
- [Google Scholar]
- Ershkov S.V., 2015. On existence of general solution of the Navier-Stokes equations for 3D non-stationary incompressible flow, Int. J. Fluid Mech. Res., 06/2015, 42 (3), pp. 206–213. Begell House.
- Ershkov S.V., 2015. Quasi-periodic non-stationary solutions of 3D Euler equations for incompressible flow. J. King Saud University – Sci., 06/2015.
- The weight function approach to uniqueness of viscous flows in unbounded domains. Arch. Rational Mech. Anal.. 1979;69(1):37-52.
- [Google Scholar]
- A quaternionic structure in the three-dimensional Euler and ideal magneto-hydrodynamics equations. Physica D. 2002;166:17-28.
- [Google Scholar]
- Quaternions and particle dynamics in the Euler fluid equations. Nonlinearity. 2006;19(8):1969-1983.
- [Google Scholar]
- Hand-book for Ordinary Differential Eq. Moscow: Science; 1971.
- Generator of solutions for 2D Navier - Stokes equations. J. Siberian Federal Univ. Math. Phys.. 2014;7(3):324-330.
- [Google Scholar]
- The Mathematical Theory of Viscous Incompressible Flow (second ed.). New York: Gordon and Breach; 1969.
- Landau, L.D., Lifshitz, E.M., 1987. Fluid Mechanics, Course of Theoretical Physics 6 (second revised ed.). Pergamon Press, ISBN 0-08-033932-8.
- An Informal Introduction to Theoretical Fluid Mechanics. Oxford University Press; 1986. ISBN 0-19-853630-5
- Theoretical Hydrodynamics. Macmillan; 1950.
- Vortex Dynamics. Cambridge University Press; 1995.
- Some new identities for solenoidal fields and applications. Mathematics (2):29-36. doi:3390/math2010029
- [Google Scholar]
- Quaternionic-valued ordinary differential equations. The Riccati equation. J. Diff. Equ.. 2009;247:2163-2187.
- [Google Scholar]







