Plane failure in rock slopes – A review on stability analysis techniques

2.1.1 Geometry of the slope

The geometry of the slope having plane mode of failure is defined by; inclination of the slope (α_f), upper slope surface inclination (α_s), slope height (h), potential failure plane – dip amount (α_p) and dip direction (Ψ_p), tension crack or upper release joint (α_t) and the lateral release surfaces (Hoek and Bray, 1981) (Fig. 1). The main driving force acting on the slope is the gravitational force which is directly proportional to the slope inclination (α_f). Steeper slope will be more susceptible for instability (Hamza and Raghuvanshi, 2017; Raghuvanshi et al., 2014). As the upper slope inclination (α_s) increases, additional rock mass will add to the weight component, thus shearing stresses will increase and instability in the slope will be induced (Sharma et al., 1995). Increase in height (h) of the slope will increase the shear stresses (Raghuvanshi et al., 2014; Anbalagan, 1992; Hack, 2002). Further, if potential failure plane (α_p) daylight on the slope face at an angle smaller to the slope face angle (α_f) and greater than the angle of friction (φ) the kinematic condition is satisfied and failure can take place (Sharma et al., 1995; Kovari and Fritz, 1984; Hoek and Bray, 1981) (Fig. 1). When the shear stress exceeds the shear strength along the potential failure surface, tension crack (α_t) develops in the upper portion of the slope. The rock mass will tend to detach along this tension crack (Sharma et al., 1995; Hoek and Bray, 1981) or release joint (Sharma et al., 1999) (Fig. 1). On the either side of the potential sliding mass there must exists lateral release surfaces which provides least resistance to the sliding mass. These release surfaces can be convergent, divergent or planer (Sharma et al., 1995; Hoek and Bray, 1981; Price, 2009) (Fig. 2).

2.1.2 Potential failure plane characteristics

The main resisting stress along the potential failure surface is defined by the shear strength parameters namely; cohesion (c) and the angle of friction (φ) (Fig. 3). The shear strength along the potential failure plane depends on the engineering geological characteristics of the discontinuity surface. These include orientation of the failure plane, continuity of the failure surface, roughness of the surface, aperture etc. (Johnson and Degraff, 1991). Assessment of these characteristics is important in defining the shear strength of the potential failure plane. The angle of friction (φ) along the potential failure plane can be estimated by law of friction proposed by Barton and Bandis (1990). The commonly used methods for estimation of ‘φ’ and ‘c’ are empirical methods and back analysis. The empirical methods are based on rock mass classification systems (Bieniawski, 1989; Hoek et al., 2002). Further, back analysis is considered to be the most reliable method to make an estimate for cohesion (c) along the potential failure plane for the anticipated conditions (Sharma et al., 1999; Singh and Goel, 2002).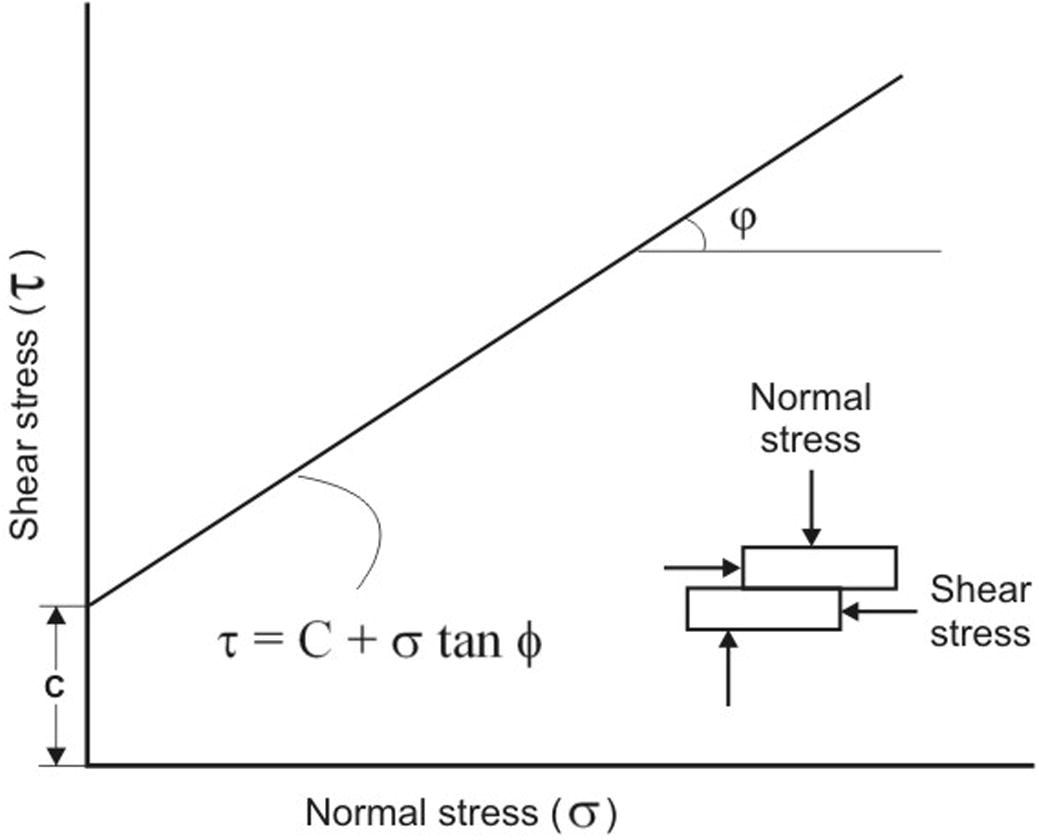

Close

Fig. 3

Relation between normal and shear stress along potential failure plane.

Export to PPT

2.1.3 Surface drainage and groundwater conditions

Surface flow on upper slope will easily find its way through the tension crack to recharge the potential failure surface. Thus, this water in tension crack will develop a water force (V) and an uplift water force (U) along the potential failure surface, this will reduce the stability of the slope (Hossain, 2011; Ahmadi and Eslami, 2011; Sharma et al., 1995; Hoek and Bray, 1981) (Fig. 4). The water along the potential failure surface will reduce the shear strength and also lubricate the surface that may facilitate the sliding process (Raghuvanshi et al., 2015; Hack, 2002). Therefore, it is very important to consider surface drainage conditions while assessing the stability of the slope.

Close

Fig. 4

Water force and pressure distribution in slope having potential plane mode of failure.

Export to PPT

2.2.1 Rainfall

Rainfall is a principal instability triggering factor in rock slopes (Ermias et al., 2017; Raghuvanshi et al., 2014; Dahal et al., 2006; Ayalew et al., 2004; Dai and Lee, 2001; Collison et al., 2000). This is evident from the fact that most of the slopes fail during rainy season (Ermias et al., 2017; Raghuvanshi et al., 2015, 2014). Surface flow on upper slope due to rainfall will easily find its way through tension crack to recharge the potential failure surface. Thus, instability in the slope will be induced.

2.2.2 Seismicity

The rock slopes under seismic loading are subjected to accelerations which induce instability in the rock slope (Ermias et al., 2017; Bommer and Rodrı’guez, 2002; Keefer, 2000). The slopes which are stable under static conditions may destabilize under dynamic seismic loading (Hoek and Bray, 1981). Under seismic loading the slope having potential plane mode of failure may easily destabilize, as one of the resolved component of the horizontal acceleration acting along the potential failure plane will add to the driving forces and the other component will be acting against the resisting forces (Fig. 5).

Close

Fig. 5

Slope subjected to horizontal acceleration during seismic loading.

Export to PPT

2.2.3 Manmade activities

Road construction is the major manmade activity on the hill slopes (Ermias et al., 2017; Wang and Niu, 2009). Very often slopes are cut in unplanned manner, leaving unsupported overhanging steep slopes. The natural slope stability is disturbed and instability in slope is induced (Tang et al., 2016). If kinematic conditions for plane mode of failure exist, the slope may easily fail. Other aspect related to manmade activities on slopes is, adding surcharge to the slope by constructing buildings and other civil engineering structures on the slopes. Such surcharge will directly add to the weight of the siding mass and if kinematic conditions exists probability for slope failure will increase (Shukla et al., 2009).

3.1.1 Kinematic method

Kinematic methods are based on the principle of kinematics which deals with the geometric condition that is required for the movement of the rock block over the discontinuity plane, without considering any forces responsible for the sliding (ZainAlabideen and Helal, 2016; Karaman et al., 2013; Kulatilake et al., 2011; Goodman, 1989). The commonly used kinematic method to determine possible mode of failure was initially proposed by Markland (1972) and later it was redefined by Hocking (1976). For kinematic check stereographic projections are used (ZainAlabideen and Helal, 2016). On a stereo-net the representative great circles for all preferred discontinuity planes, present on the given slope, are plotted. Also, great circle for slope face and friction circle, corresponding to the friction angle of the discontinuity plane, are plotted. The zone demarcated by the friction circle and the slope face is designated as sliding envelope (Fig. 6). If any great circle of a discontinuity plane, having strike nearly parallel to the slope face, falls within this sliding envelope, kinematic condition is satisfied (Ali et al., 2015).

Close

Fig. 6

Kinematic condition for plane mode of failure.

Export to PPT

Hoek and Bray (1981) further redefined the kinematic condition for plane mode of failure by introducing two more general conditions; (i) the strike difference between the slope face and the potential failure surface must be nearly parallel (±20°) and (ii) there must be lateral release surfaces on either sides of the sliding block which must not provide any resistance to the sliding. Price (2009) classified these lateral boundaries as convergent, divergent and planar (Fig. 2). Further, Yoon et al. (2002) divided lateral release surfaces into two types; (i) when discontinuity intersect the potential failure plane and (ii) when free faces intersects the slope face. The lateral release surfaces may lead to single faced slope (SFS) sliding or multi faced slope (MFS) sliding. Slopes which are MFS show varied sliding conditions owing to complex slope geometric characteristics. Yoon et al. (2002) suggested kinematic check for such multi faced slopes. Further, Lisle (2004) presented equations to calculate daylight envelope for plane failure in rock slopes. Kinematic check is the first step to proceed for other analytical techniques.

3.1.2 Empirical methods

In past several empirical methods based on rock mass classification systems have been developed. The important slope classification systems are; classification proposed by Selby (1980), Slope Mass Rating (SMR) (Romana, 1985), Modified Slope Mass Rating (MSMR) (Anbalagan et al., 1992), Slope Stability Probability Classification (SSPC) (Hack, 1998) and rock mass classification system for slopes proposed by Liu and Chen (2007).

SMR classification, proposed by Romana (1985) can be used to assess the stability condition of a rock slope (Alzo’ubi, 2016). SMR utilizes Bieniawski’s (1979) rock mass rating (RMR), the relationship between parallelism of slope and discontinuities, dip amount of the discontinuity and relation between the slope inclination and dip of the discontinuity. Also, mode of excavation is considered in SMR. Anbalagan et al. (1992) modified SMR by considering wedge mode of failure as a separate case. For stability analysis of slope, having plane mode of failure, both SMR and MSMR classifications can be utilized. Hack (1998) proposed SSPC to classify the rock mass and to define its in situ stability condition with probability of failure to occur. The SSCP accounts for discontinuity relations with the slope, degree of weathering and the shear strength of the slope material (Singh and Goel, 2002). In SSPC the exposed rock mass is characterized to represent in its imaginary un-weathered and undisturbed state for which suitable corrections for weathering and excavation disturbance are made (Karaman et al., 2013; Hack, 1998). Further, Liu and Chen (2007) proposed a classification system for the assessment of rock slope stability. In this classification geological, geometric and environmental factors were considered. By combining Fuzzy Delphi method and Analytic Hierarchy Process a model to estimate the rock mass quality was developed.

3.1.3 Limit equilibrium methods

Limit equilibrium methods are relatively simple in its application, as compared to the numerical methods (Tang et al., 2016; Yang and Zou, 2006; Eberhardt et al., 2004). For plane mode of failure analysis, the limit equilibrium methods were proposed by many researchers, such as; Tang et al. (2016), Ahmadi and Eslami (2011), Shukla et al. (2009), Price (2009), Hoek (2007), Zheng et al. (2005), Sharma et al. (1999), Ling and Cheng (1997), Sharma et al. (1995), Hoek and Bray (1981) etc. In limit equilibrium method various forces responsible for driving of the rock mass and the resisting forces are evaluated and the ratio of resisting forces to driving forces at equilibrium, defines the factor of safety (FOS) (Price, 2009; Sharma et al., 1995; Hoek and Bray, 1981). If FOS value is more than ‘1’ it suggest stable slope, if less than ‘1’ unstable and if FOS is equal to ‘1’ the slope is in a critical state of equilibrium (Hossain, 2011). The FOS will be affected by various governing factors such as; geometry of the slope, failure plane characteristics, water forces and external triggering factors. The FOS is inversely proportional to the height of the slope. Increase in height (h) of the slope will increase the shear stresses, thus FOS will decrease (Raghuvanshi et al., 2014; Anbalagan, 1992; Hoek and Bray, 1981; Hack, 2002; Raghuvanshi and Solomon, 2005). Steeper slope angle will directly affect the FOS. The main driving force acting on the slope is the gravitational force which is directly proportional to the slope inclination (Hamza and Raghuvanshi, 2017; Raghuvanshi et al., 2014). Similarly, increase in upper slope inclination will increase FOS (Sharma et al., 1995). Also, the FOS is directly proportional to the shear strength of the potential failure plane (Hoek and Bray, 1981). The shear strength of the potential failure plane is defined by parameters; cohesion (c) and angle of friction (φ). As the shear strength increases, FOS will increase.

Hoek and Bray (1981) provided a 2D analytical solution for plane mode of failure. For this method the required general conditions are; (i) the dip of the potential failure plane should be less than the slope inclination (ii) the dip of the potential failure plane must be greater than the angle of friction, (iii) the strike of the potential failure plane must be nearly parallel (±20°) to the slope and (iv) there must be lateral boundaries on either side of the sliding block which place negligible resistance to the sliding mass. Besides, few assumptions were also made by Hoek and Bray (1981). The geometry of the slope used in this analytical solution is presented in Fig. 7. In Hoek and Bray (1981) technique it was assumed that tension crack is vertical and the upper slope is horizontal. Later, Sharma et al. (1995) explained that upper slope is seldom horizontal for natural slopes and in most of the cases it is inclined. They also showed that the value of FOS decreases considerably when the upper slope surface angle is increased. Similarly, the tension crack may not always be vertical. Thus, Sharma et al. (1995) modified the Hoek and Bray’s (1981) analytical solution by considering the actual upper slope inclination and non vertical tension crack (Fig. 7). Further, Ling and Cheng (1997) proposed an analytical method for the slope stability assessment. The rock mass was assumed to be a rigid body having plain strain condition. For this analytical formulation, forces due to the weight of the sliding mass, water forces and the seismic coefficients were considered. Later, Sharma et al. (1999) proposed analytical solution for plane mode of failure in which they redefined the tension crack as a release-joint which may be a pre-existing discontinuity. Shukla et al. (2009) also presented an analytical solution for the plane failure analysis by considering factors such as; the weight of the sliding mass, water forces in the tension crack and along the potential failure plane, shear strength parameters of the potential failure plane, slope inclination, surcharge load and the seismic factors. Further, Ahmadi and Eslami (2011) proposed an analytical solution in which the effect of water forces was studied.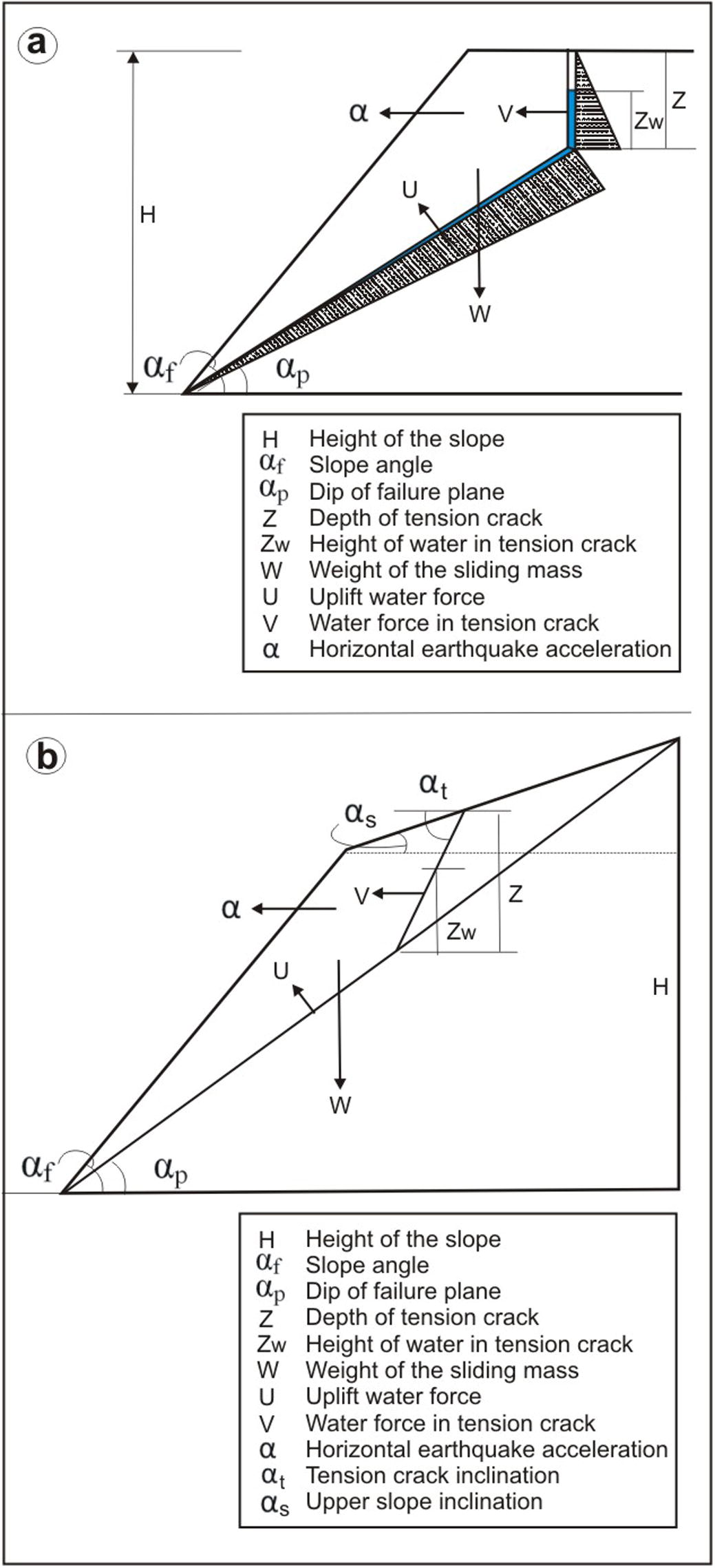

Close

Fig. 7

Geometry of slope and various force vectors used in (a) Hoek and Bray (1981) analytical technique (b) Sharma et al. (1995) modified technique.

Export to PPT

3.1.4 Probabilistic methods

The probabilistic methods facilitate to incorporate parameters, which show uncertainty, in a systematic way and define the stability condition of the slope in probabilistic terms (Alzo’ubi, 2016; Chowdhury, 2010). For probabilistic analysis of a slope, having plane mode of failure, the parameters to be used are first defined as fixed dimension parameters and as random variables (Hoek, 2007). Fixed dimension parameters are mainly the geometric parameters which can be obtained directly from the geometry of the slope such as; slope height, slope inclination, upper slope inclination and dip of the potential failure plane. The random variables are those which show uncertainty in their values and may vary considerably such as; cohesion (c) and angle of friction (φ), ratio of depth of water in tension crack to the depth of the tension crack etc. (Hoek, 2007). FOS is the ratio between the resisting forces and the driving forces. As earlier stated, since some of the parameters used in resisting and driving forces are random variables therefore, such parameters will have probability distribution over certain range, rather than a fixed absolute value. Thus, the probabilistic analysis will also provide FOS as random variables with probability distribution (Chowdhury, 2010).

In probabilistic framework for plane failure analysis, three methods are followed (i) First Order Second Moment approach (FOSA), (ii) Point estimate approach (PEA) and (iii) Monte Carlo Simulation approach (MSA). The FOSA provide expected value of FOS and its variance. In PEA method the discrete values of FOS can be estimated at the mean values of the variables (Chowdhury, 2010). Through PEA, mean and standard deviation of FOS can be computed when input parameters show random behavior (Hoek, 2007). In MSA method from the probability distribution of each variable, discrete values are randomly selected. Later, FOS is evaluated by utilizing a set of different discrete values of various parameters. Multiple simulations are made by repeating the process by taking different set of the discrete values of various variables (Zhao et al., 2016; Chowdhury, 2010).

3.2.1 Continuum modeling

The continuum modeling approach can be applied to those rock slopes where the rock mass is relatively uniform such as; heavily disintegrated rock mass which can be considered as continuous all over the slope. The variability of various governing parameters can be simulated in the stability analysis for the static and dynamic conditions. The continuum modeling is based on finite element, finite difference and boundary element methods. The basic input data that is required for continuum modeling approach are; constitutive model, in-situ stress, shear strength parameters for surfaces, groundwater etc. For the slope stability analysis through continuum modeling finite difference and finite element models are commonly used. These methods can suitably be applied for weak rock masses in which failure results due to intact rock deformation or through the discrete stratified discontinuities (Stead et al., 2006). The major limitation with continuum techniques is in defining rock mass as continuum, for which simplifications are needed. Thus, the final results are highly influenced by such simplifications (Hack, 2002).

3.2.2 Discontinuum modeling

The discontinuum modeling is suitable for discontinuous rock mass which contains discontinuities and the failure mechanism is controlled by pre-existing discontinuities. In discontinuum analysis movement of intact rock blocks bounded within discontinuities and the deformation of intact rock can be addressed for both, static and the dynamic conditions (Stead et al., 2006). The input data required for modeling includes data on slope geometry, discontinuity characteristic, discontinuity shear strength and stiffness, in-situ stress and groundwater data. For discontinuum modeling, distinct element method and discontinuous deformation analysis are widely used. For plane failure mechanism distinct element method can be used (Stead et al., 2006). The discontinuum method is quite effective to simulate the discontinuity behavior and to assess the rock slope stability for given conditions.

3.2.3 Hybrid modeling

Hybrid modeling approaches utilizes the combined capabilities of both continuum and discontinuum methods. The strength of the rock mass is a combined effect of strength along the rock joints and the strength of the intact rock between the joints (Alzo’ubi, 2016). Complex slope and discontinuity geometry can be considered in hybrid modeling approach. Step-path geometries can be simulated by hybrid finite-discrete element method with fracture propagation approach (Stead et al., 2006).