Translate this page into:
On Skolem odd and even difference mean graphs
⁎Corresponding author. geeclau@yahoo.com (Gee-Choon Lau),
-
Received: ,
Accepted: ,
This article was originally published by Elsevier and was migrated to Scientific Scholar after the change of Publisher.
Peer review under responsibility of King Saud University.
Abstract
Let be a simple, finite and undirected -graph with p vertices and q edges. A graph G is Skolem odd difference mean if there exists an injection and an induced bijection such that each edge uv (with ) is labeled with . We say G is Skolem even difference mean if there exists an injection and an induced bijection such that each edge uv (with ) is labeled with . A graph that admits a Skolem odd (or even) difference mean labeling is called a Skolem odd (or even) difference mean graph. In this paper, first, we construct some new Skolem odd difference mean graphs and then investigate the Skolem even difference meanness of some standard graphs.
Keywords
Mean labeling
Odd mean labeling
Skolem difference mean labeling
Skolem odd difference mean labeling
Skolem even difference mean labeling
05C78

1 Background
Let be a simple, finite and undirected -graph of order and size . A graph labeling is an assignment of integers to the vertices or edges (or both) of a graph subject to certain conditions. Many types of labeling have been introduced over the last few decades. An excellent survey of graph labeling is available in Gallian (2016). Terms and notations not defined here are used in the sense of Harary (1972). The concept of mean graph was introduced in Somasundaram and Ponraj (2003). A graph G is called a mean graph if there is an injection such that each edge uv is labeled with and the resulting edge labels are distinct. In 2006, Manickam and Marudai studied the odd mean labeling of graphs. A graph G is said to be odd mean if there exists an injection and an induced bijection such that edge uv is labeled with . The notion of Skolem difference mean graph was due to Murugan and Subramanian (2011) and further studied by Ramya et al. (2013) and Ramya and Selvi (2014). A graph is difference mean if there exists an injection and an induced bijection such that each edge uv (with ) is labeled with . Ramya et al. (2014) defined the concept of Skolem odd difference mean graph and further studied in Jeyanthi et al. (2016). A graph G is Skolem odd difference mean if there exists an injection and an induced bijection such that each edge uv (with ) is labeled with . Moreover, G is a Skolem even vertex odd difference mean graph if for each vertex is even. Motivated by the results in Ramya et al. (2014) and Jeyanthi et al. (2016), in this paper, we introduce the dual version of Skolem odd difference mean labeling. A graph G is Skolem even difference mean if there exists an injection and an induced bijection such that each edge uv (with ) is labeled with . Moreover, G is a Skolem even vertex even difference mean graph if for each vertex is even. In the present study, we also use the following definitions.
Let be the complete bipartite graph with partite sets of size m and n respectively. A caterpillar is obtained from a path by adding pendant edges to vertex . The coconut tree is obtained by identifying the central vertex of the star with a pendant vertex of a path . The graph is obtained from and m copies of by identifying one pendant vertex of the i-th copy of with the i-th vertex of . The graph is the disjoint union of m copies of . Let be the bistar obtained from a and a by joining their central vertex u and v with an edge. The graph , is obtained from the bistar by replacing the edge uv with . A graph obtained from a path by attaching exactly two pendant edges to each internal vertex of a path , is called a twig and is denoted by .
This study is organized into three sections. In Section 2, we show that there exist Skolem odd difference mean graphs with non-cycle and non-tree component(s). In Section 3, we investigate the Skolem even difference meanness of some standard graphs.
2 Construction of Skolem odd difference mean graphs
By definition, it is a fact that if G is a Skolem odd difference mean -graph, then (also see Theorem 2.6 in Selvi et al. (2015) for a proof by contrapositive). If G is connected, then or . Hence, G is a graph with one cycle or a tree.
If G is a connected Skolem odd difference mean -graph, then is odd for some .
By definition, which is odd. Hence the largest edge label must be obtained by labeling two adjacent vertices with 0 and giving us an odd vertex label. □
The disjoint union of paths of length at least 2 is a Skolem even vertex odd difference mean graph.
Suppose the paths are and for . Let the vertices of the i-th path be to . Now we have vertices and edges. Hence, we need to label the vertices by integers in .
-
Label by for (or if is even).
-
For , let be the largest used label for . Label of by for (or if is even).
-
Label by for (or if is odd).
-
For , let be the largest used label for . Label of by for (or if is odd).
It is easy to verify that the largest vertex label used is . Moreover, all the vertex labels are even such that the induced edge labels are odd integers from 1 to . □
For example, we can label the vertices of by according to the labeling function as defined above.
The graph is a Skolem odd difference mean graph for and .
Consider . Let . Define a function by for , and for .
The induced edge label function is defined as , and for .
Consider . Let . Define a function by for , and for .
The induced edge label function is defined as , for . Thus, f is a Skolem odd difference mean labeling of for and . □
The graph is a Skolem even vertex odd difference mean graph for all .
Let the vertices of be and whereas the vertices of be so that the edges are and . Define a function as and (see Fig. 1).
The induced edge label function is defined as and for . Thus f is a Skolem even vertex odd difference mean labeling of . □
The graph is Skolem odd difference mean for with all vertex labels of same parity.
If G is a Skolem even vertex odd difference mean -graph, then is a Skolem odd difference mean graph for all .
Let G be a Skolem even vertex odd difference mean -graph with a labeling and its induced edge labeling . The edge set labels are . Define with and so that and . Define an injective function such that for each . Similarly to the vertex labeling of for Theorem 2.4, also define for . The induced edge label function is defined as and for . Thus, are odd and . Hence g is a Skolem odd difference mean labeling of . □
The graph is a Skolem odd difference mean graph if G is a Skolem even vertex odd difference mean graph as in Theorems 2.2 and 2.3 or in Somasundaram and Ponraj (2003).
If G is a Skolem even vertex odd difference mean -graph, then is a Skolem odd difference mean graph for all .
Let G be a Skolem even vertex odd difference mean -graph with a labeling and its induced edge labeling . The edge set labels are . Define with and so that and . Define an injective function such that for each for and for . The induced edge label function is defined as and for . It can be verified that is odd for , and . Hence g is a Skolem odd difference mean labeling of for all . □
The graph is a Skolem odd difference mean graph if G is a Skolem even vertex odd difference mean graph as in Theorems 2.2 and 2.3 or in Somasundaram and Ponraj (2003).
If G is a Skolem even vertex odd difference mean -graph, then is a Skolem odd difference mean graph for all .
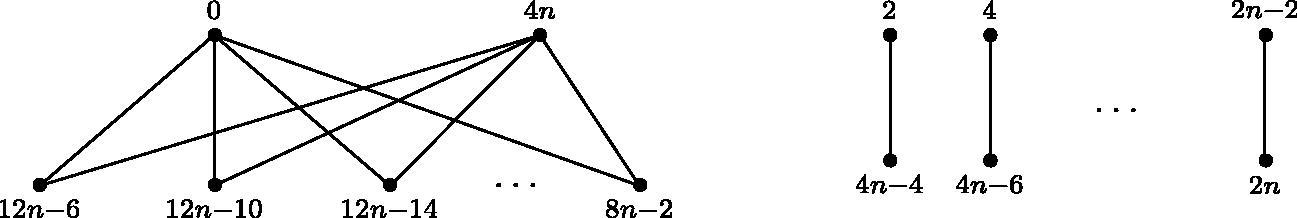
Let G be a Skolem even vertex odd difference mean -graph with a labeling and its induced edge labeling . The edge set labels are . Define with and so that and . Define an injective function such that for each and for (see Fig. 2).
The induced edge label function is defined as and for . It can be verified that is odd for , and . Hence g is a Skolem odd difference mean labeling of for all . □
The graphs are Skolem odd difference mean graph if G is a Skolem even vertex odd difference mean graph as in Theorems 2.2 and 2.3 or in Somasundaram and Ponraj (2003).
- Vertex labeling of
.

- Vertex labeling of
in
.
3 Skolem even difference mean graphs
As a natural extension, we introduce in this section the Skolem even difference mean labeling of graphs.
If G is a Skolem even difference mean -graph, then (similar to Theorem 2.6 in Selvi et al. (2015)).
If , then any Skolem even difference mean labeling of G must admit an even vertex labeling.
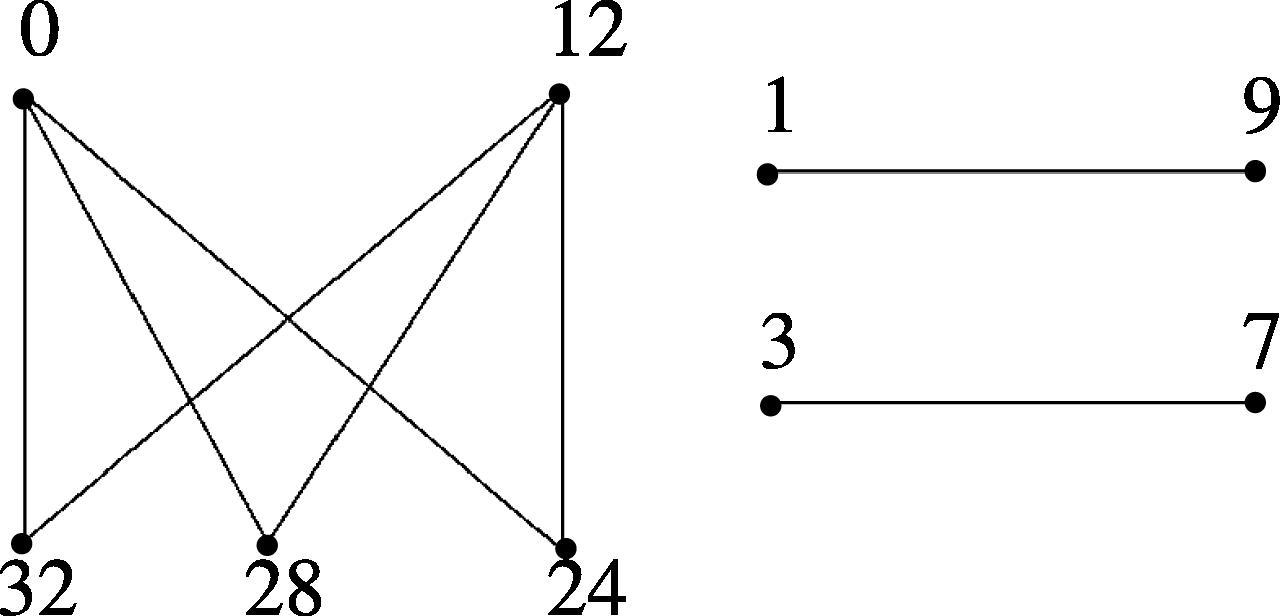
The caterpillar is a Skolem even vertex even difference mean graph.
Let and . Define a function such that
-
for ,
-
for ,
-
for , and for ,
-
for , and for .
Let for and for . For each vertex label f the induced edge label is defined as follows: for , for .
Thus f is a Skolem even difference mean labeling of . Hence is a Skolem even difference mean graph. □
For example, the Skolem even difference mean labeling of is shown in Fig. 3.
The graphs (i) , (ii) and (iii) are Skolem even difference mean.
It follows from Theorem 3.1 such that for (i), we take ; for (ii), we take ; for (iii), we take and . □

-
is a Skolem even difference mean graph.
The following examples illustrate the three cases in the corollary.
-
Case (i).
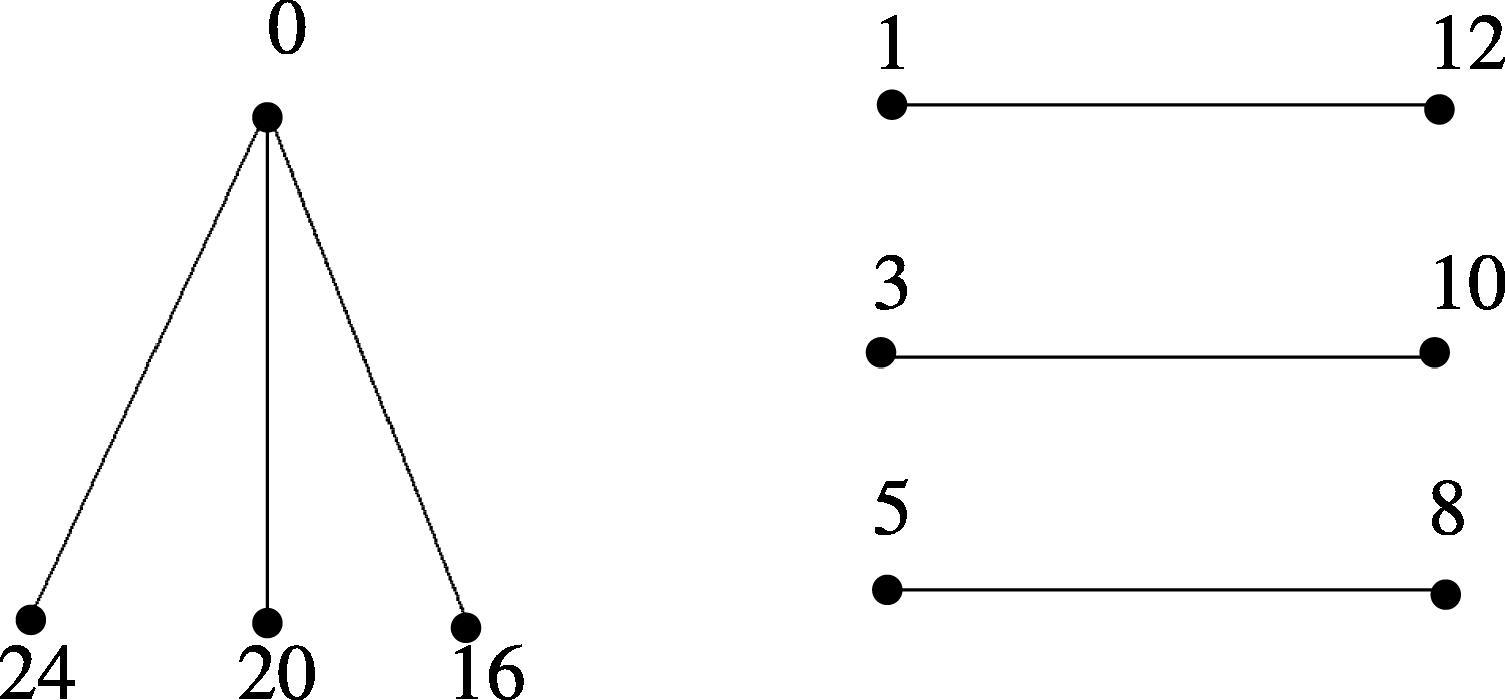
The Skolem even difference mean labeling of is shown in Fig. 4.
-
Case (ii).
The Skolem even difference mean labeling of is shown in Fig. 5.
-
Case (iii).
The Skolem even difference mean labeling of is shown in Fig. 6.
The graph is a Skolem even difference mean graph.
Let and . Define a function such that
Let for and for . For each vertex label of f, the induced edge label function is defined as follows:
Thus f is a Skolem even difference mean labeling of . □

-
is a Skolem even difference mean graph.
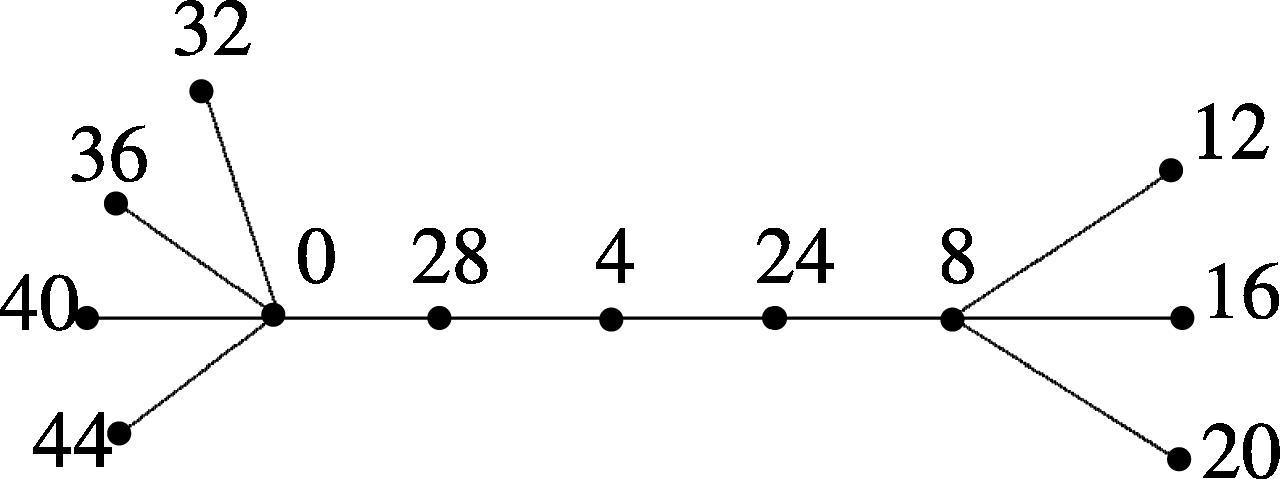
-
is a Skolem even difference mean graph.
-
is a Skolem even difference mean graph.
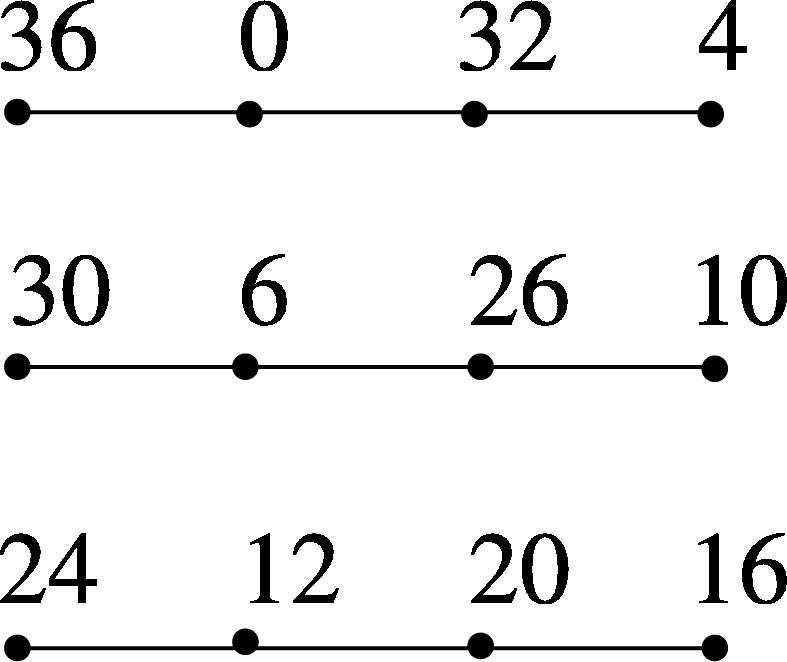
The Skolem even difference mean labeling of is shown in Fig. 7.
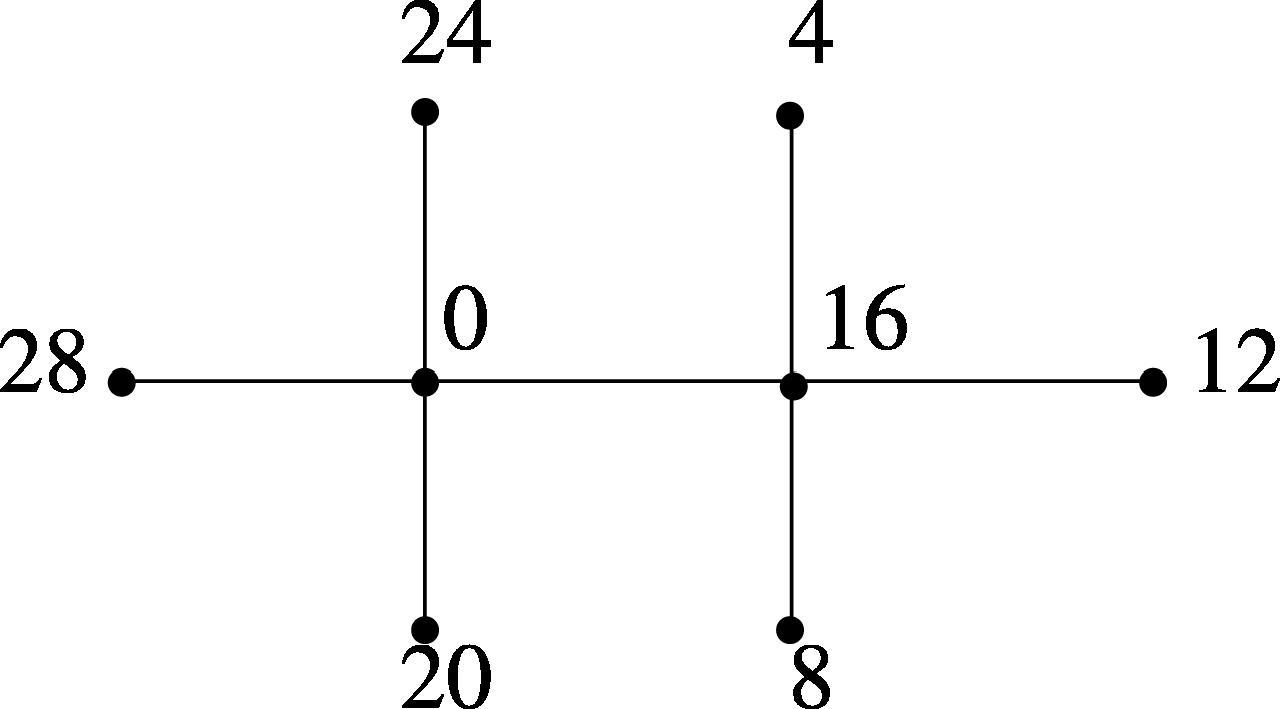
The graph is a Skolem even difference mean graph.
Let and . Define a function as follows:
If n is odd, then
If n is even, then
For each vertex labeling of f, the induced edge label function is defined as for . Thus f is a Skolem even difference mean labeling of .
-
is a Skolem even difference mean graph.
The Skolem even difference mean labeling of is shown in Fig. 8.
If G is a Skolem even vertex even difference mean -graph, then is a Skolem even difference mean graph for all .
Let G be a Skolem even vertex even difference mean -graph with labeling and its induced edge labeling function . Clearly, is even for each and . Define with and so that and . Define an injective function such that for each and for . The induced edge label function is defined as and for . Thus, are even and . Hence, g is a Skolem even difference mean labeling of . □
-
is a Skolem even difference mean graph.
By an argument similar to that in Theorems 2.8 and 2.10, we have the following corollary.
If G is a Skolem even vertex even difference mean -graph, then and are Skolem even difference mean graph.
The graph is a Skolem even difference mean graph for all .
Let and . Define a function such that
-
for ,
-
for ,
-
for ,
-
for .
The induced edge label function is defined as follows: for , and for . Thus, f is a Skolem even difference mean labeling of . □
The Skolem even difference mean labeling of is shown in Fig. 9.
The graph is a a Skolem even difference mean graph graph for all .
Let and . Define a function such that for for , and for . The induced edge label function is defined as for , and for . Thus, f is a Skolem even difference mean labeling of . □
-
is a Skolem even difference mean graph.
The Skolem even difference mean labeling of is shown in Fig. 10.
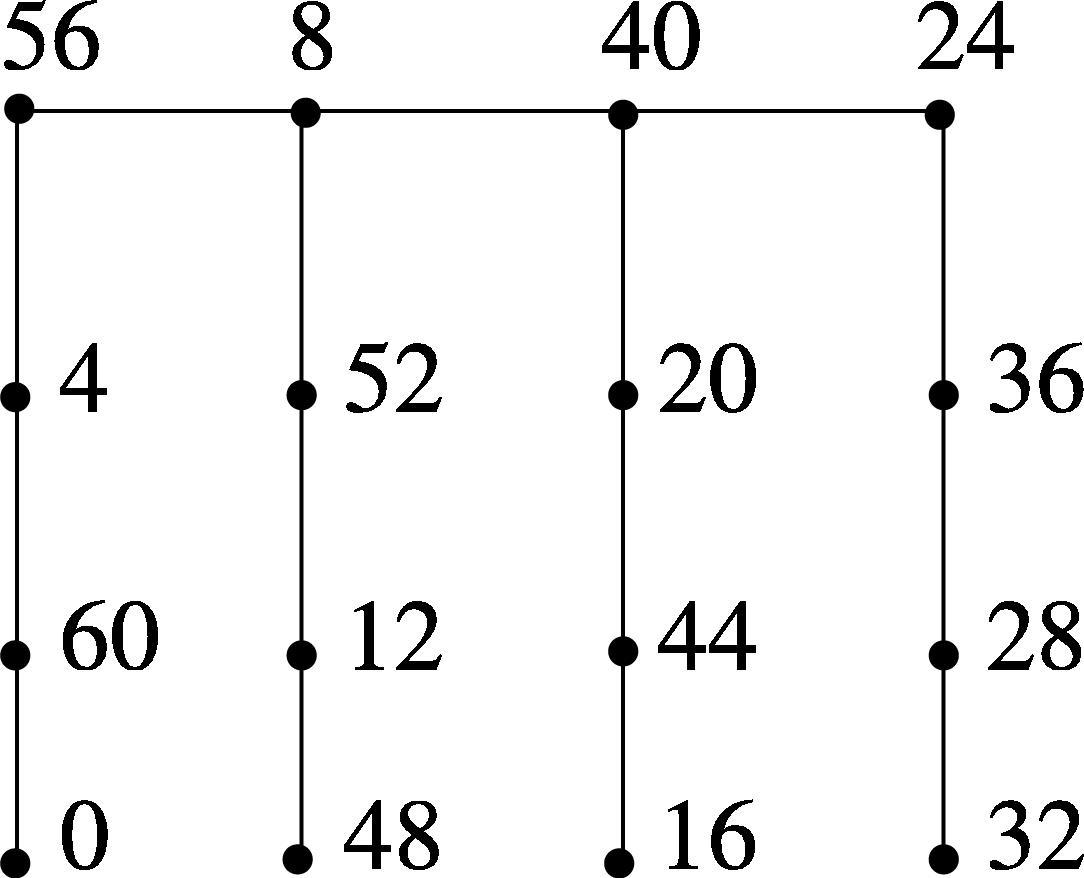
The graph is a Skolem even difference mean graph for all .
Let and . Define a function such that for for for . The induced edge label function is defined as follows:
-
,
-
for ,
-
for ,
-
,
-
for .
Thus f is a Skolem even difference mean labeling of . □
-
is a Skolem even difference mean graph.
The Skolem even difference mean labeling of
is shown in Fig. 11.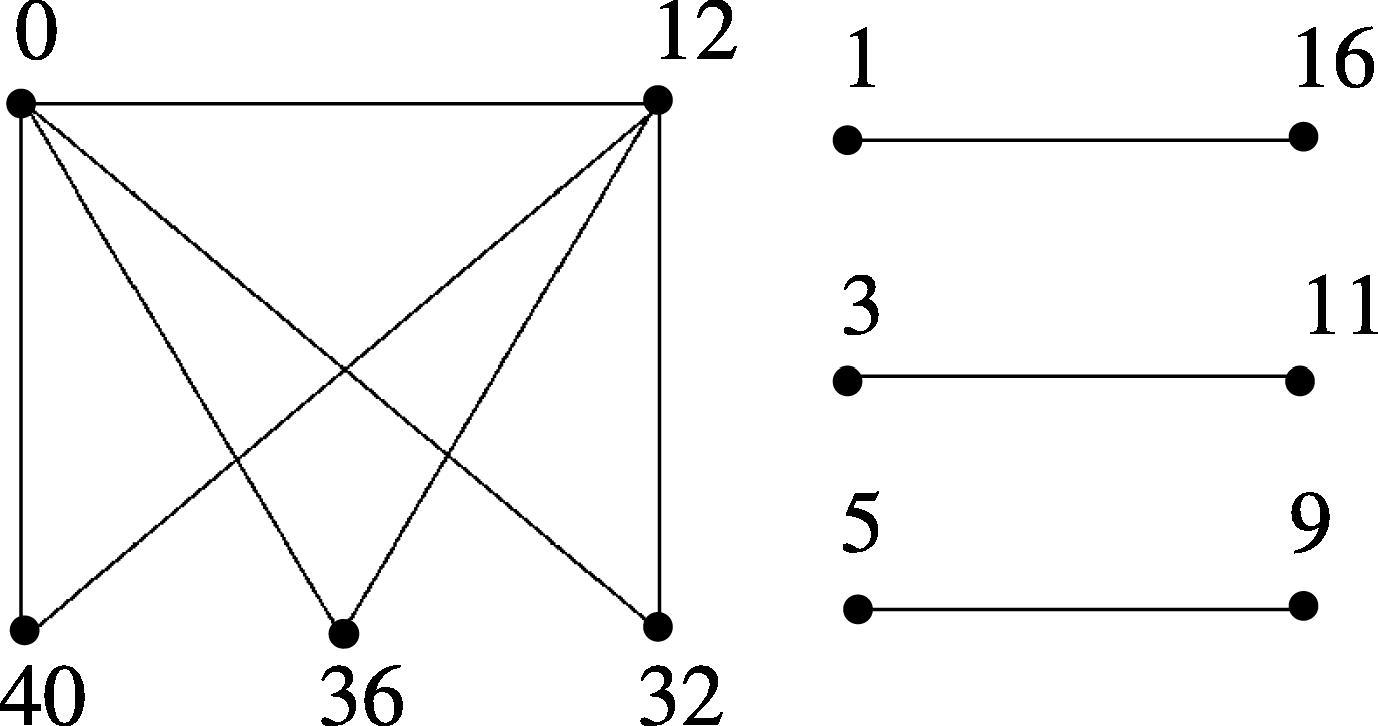
is a Skolem even difference mean graph.
4 Conclusion
In this paper first we show that there exist Skolem odd difference mean labeling for graphs with non-cycle and non-tree components. Further, we introduce the concept of Skolem even difference mean labeling. We conclude the paper with the following open problem.
Establish the Skolem even difference mean labeling of where G is a (complete) multipartite graph and .
Acknowledgements
The authors sincerely thank the reviewers for their constructive suggestions that help to improve the presentation of this paper to a great extent.
References
- Gallian, J., 2016. A Dynamic Survey of Graph Labeling, Electron. J. Combin. # DS 6.
- Graph theory. Massachusetts, USA: Addison Wesley; 1972.
- Some results on skolem odd difference mean labeling. Proyecciones J. Maths.. 2016;35(4):407-417.
- [Google Scholar]
- Skolem difference mean labeling of H-graphs. Int. J. Math. Soft Comput.. 2011;1(1):115-129.
- [Google Scholar]
- On Skolem difference mean labeling of graphs. Int. J. Math. Arch.. 2013;4(12):73-79.
- [Google Scholar]
- On Skolem difference mean labeling of some trees. Int. J. Math. Soft Comput.. 2014;4(2):11-15.
- [Google Scholar]






